No CrossRef data available.
Article contents
A dilemma for reasons additivity
Published online by Cambridge University Press: 27 December 2021
Abstract
This paper presents a dilemma for the additive model of reasons. Either the model accommodates disjunctive cases in which one ought to perform some act
 $$\phi $$
just in case at least one of two factors obtains, or it accommodates conjunctive cases in which one ought to
$$\phi $$
just in case at least one of two factors obtains, or it accommodates conjunctive cases in which one ought to
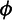 $$\phi $$
just in case both of two factors obtains. The dilemma also arises in a revised additive model that accommodates imprecisely weighted reasons. There exist disjunctive and conjunctive cases. Hence the additive model is extensionally inadequate. The upshot of the dilemma is that one of the most influential accounts of how reasons accrue to determine what we ought to do is flawed.
$$\phi $$
just in case both of two factors obtains. The dilemma also arises in a revised additive model that accommodates imprecisely weighted reasons. There exist disjunctive and conjunctive cases. Hence the additive model is extensionally inadequate. The upshot of the dilemma is that one of the most influential accounts of how reasons accrue to determine what we ought to do is flawed.
- Type
- Article
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press



