Article contents
An Abramov formula for stationary spaces of discrete groups
Published online by Cambridge University Press: 15 January 2013
Abstract
Let 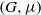 $(G, \mu )$ be a discrete group equipped with a generating probability measure, and let
$(G, \mu )$ be a discrete group equipped with a generating probability measure, and let  $\Gamma $ be a finite index subgroup of
$\Gamma $ be a finite index subgroup of 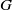 $G$. A
$G$. A 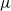 $\mu $-random walk on
$\mu $-random walk on 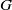 $G$, starting from the identity, returns to
$G$, starting from the identity, returns to 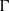 $\Gamma $ with probability one. Let
$\Gamma $ with probability one. Let  $\theta $ be the hitting measure, or the distribution of the position in which the random walk first hits
$\theta $ be the hitting measure, or the distribution of the position in which the random walk first hits  $\Gamma $. We prove that the Furstenberg entropy of a
$\Gamma $. We prove that the Furstenberg entropy of a 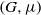 $(G, \mu )$-stationary space, with respect to the action of
$(G, \mu )$-stationary space, with respect to the action of 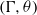 $(\Gamma , \theta )$, is equal to the Furstenberg entropy with respect to the action of
$(\Gamma , \theta )$, is equal to the Furstenberg entropy with respect to the action of 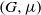 $(G, \mu )$, times the index of
$(G, \mu )$, times the index of 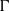 $\Gamma $ in
$\Gamma $ in 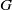 $G$. The index is shown to be equal to the expected return time to
$G$. The index is shown to be equal to the expected return time to 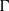 $\Gamma $. As a corollary, when applied to the Furstenberg–Poisson boundary of
$\Gamma $. As a corollary, when applied to the Furstenberg–Poisson boundary of 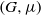 $(G, \mu )$, we prove that the random walk entropy of
$(G, \mu )$, we prove that the random walk entropy of 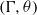 $(\Gamma , \theta )$ is equal to the random walk entropy of
$(\Gamma , \theta )$ is equal to the random walk entropy of 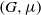 $(G, \mu )$, times the index of
$(G, \mu )$, times the index of 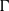 $\Gamma $ in
$\Gamma $ in 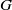 $G$.
$G$.
Information
- Type
- Research Article
- Information
- Copyright
- ©2013 Cambridge University Press
References
- 4
- Cited by

