Article contents
Enveloping semigroups and quasi-discrete spectrum
Published online by Cambridge University Press: 15 June 2015
Abstract
The structures of the enveloping semigroups of certain elementary finite- and infinite-dimensional distal dynamical systems are given, answering open problems posed in 1982 by Namioka [Ellis groups and compact right topological groups. Conference in Modern Analysis and Probability (New Haven, CT, 1982) (Contemporary Mathematics, 26). American Mathematical Society, Providence, RI, 1984, 295–300]. The universal minimal system with (topological) quasi-discrete spectrum is obtained from the infinite-dimensional case. It is proved that, on the one hand, a minimal system is a factor of this universal system if and only if its enveloping semigroup has quasi-discrete spectrum and that, on the other hand, such a factor need not have quasi-discrete spectrum in itself. This leads to a natural generalization of the property of having quasi-discrete spectrum, which is named the 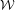 ${\mathcal{W}}$ -property.
${\mathcal{W}}$ -property.
Information
- Type
- Research Article
- Information
- Copyright
- © Cambridge University Press, 2015
References
- 3
- Cited by

