Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-14T06:24:13.733Z Has data issue: false hasContentIssue false
  • English
  • Français

Interval projections of self-similar sets

Published online by Cambridge University Press:  14 June 2018

ÁBEL FARKAS
ÁBEL FARKAS*
Affiliation:
Einstein Institute of Mathematics, Edmond J. Safra Campus, The Hebrew University of Jerusalem, Givat Ram, Jerusalem, 9190401, Israel email thesecondabel@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

We show if $K$ is a self-similar $1$-set that either satisfies the strong separation condition or is defined via homotheties then there are at most finitely many lines through the origin such that the projection of 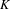$K$ onto them is an interval.

Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 40 , Issue 1 , January 2020 , pp. 194 - 212
Copyright
© Cambridge University Press, 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Besicovitch, A. S.. On the fundamental geometrical properties of linearly measurable plane sets of points (III). Math. Ann. 116 (1939), 349–357.Google Scholar
Falconer, K.. Techniques in Fractal Geometry. John Wiley & Sons, Chichester, 1997.Google Scholar
Falconer, K. and Fraser, J. M.. The visible part of plane self-similar sets. Proc. Amer. Math. Soc. 141 (2013), 269–278.10.1090/S0002-9939-2012-11312-7Google Scholar
Farkas, Á.. Projections of self-similar sets with no separation condition. Israel J. Math. 214 (2016), 67–107.Google Scholar
Federer, H.. The (𝜑, k) rectifiable subsets of n-space. Trans. Amer. Math. Soc. 62 (1947), 114–192.Google Scholar
Hutchinson, J. E.. Fractals and self-similarity. Indiana Univ. Math. J. 30 (1981), 713–747.Google Scholar
Kenyon, R.. Projecting the one-dimensional Sierpinski gasket. Israel J. Math. 97 (1997), 221–238.Google Scholar
Marstrand, J. M.. Some fundamental geometrical properties of plane sets of fractional dimensions. Proc. Lond. Math. Soc. (3) 4 (1954), 257–302.Google Scholar
Mattila, P.. Hausdorff dimension, orthogonal projections and intersections with planes. Ann. Acad. Sci. Fenn. Ser. A 1 (1975), 227–244.Google Scholar
Mattila, P.. Pertti On the structure of self-similar fractals. Ann. Acad. Sci. Fenn. Ser. A 7 (1982), 189–195.Google Scholar
Mattila, P.. Geometry of Sets and Measures in Euclidean Spaces. Cambridge University Press, Cambridge, 1995.Google Scholar
Schief, A.. Separation properties for self-similar sets. Proc. Amer. Math. Soc. 122 (1994), 111–115.10.1090/S0002-9939-1994-1191872-1Google Scholar
Wang, J. L.. The open set conditions for graph directed self-similar sets. Random Comput. Dynam. 5 (1997), 283–305.Google Scholar