No CrossRef data available.
Article contents
Topologically irreducible representations of the Banach  $\ast$ -algebra associated with a dynamical system
$\ast$ -algebra associated with a dynamical system
Published online by Cambridge University Press: 24 January 2017
Abstract
We describe (infinite-dimensional) irreducible representations of the crossed product C $^{\ast }$ -algebra associated with a topological dynamical system (based on
$^{\ast }$ -algebra associated with a topological dynamical system (based on  $\mathbb{Z}$ ) and we show that their restrictions to the underlying
$\mathbb{Z}$ ) and we show that their restrictions to the underlying 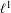 $\ell ^{1}$ -Banach
$\ell ^{1}$ -Banach  $\ast$ -algebra are not algebraically irreducible under mild conditions on the dynamical system. The above description of irreducible representations has two ingredients, ergodic measures on the space and ergodic extensions for the tensor product with type I factors, the latter of which may not have been explicitly taken up before but which will be explored by means of examples. A new class of ergodic measures is also constructed for irrational rotations on the circle.
$\ast$ -algebra are not algebraically irreducible under mild conditions on the dynamical system. The above description of irreducible representations has two ingredients, ergodic measures on the space and ergodic extensions for the tensor product with type I factors, the latter of which may not have been explicitly taken up before but which will be explored by means of examples. A new class of ergodic measures is also constructed for irrational rotations on the circle.
Information
- Type
- Original Article
- Information
- Copyright
- © Cambridge University Press, 2017

