Article contents
Central sets generated by uniformly recurrent words
Published online by Cambridge University Press: 11 October 2013
Abstract
A subset 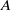 $A$ of
$A$ of 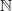 $ \mathbb{N} $ is called an IP-set if
$ \mathbb{N} $ is called an IP-set if 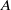 $A$ contains all finite sums of distinct terms of some infinite sequence
$A$ contains all finite sums of distinct terms of some infinite sequence 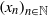 $\mathop{({x}_{n} )}\nolimits_{n\in \mathbb{N} } $ of natural numbers. Central sets, first introduced by Furstenberg using notions from topological dynamics, constitute a special class of IP-sets possessing rich combinatorial properties: each central set contains arbitrarily long arithmetic progressions and solutions to all partition regular systems of homogeneous linear equations. In this paper we investigate central sets in the framework of combinatorics on words. Using various families of uniformly recurrent words, including Sturmian words, the Thue–Morse word and fixed points of weak mixing substitutions, we generate an assortment of central sets which reflect the rich combinatorial structure of the underlying words. The results in this paper rely on interactions between different areas of mathematics, some of which have not previously been directly linked. They include the general theory of combinatorics on words, abstract numeration systems, and the beautiful theory, developed by Hindman, Strauss and others, linking IP-sets and central sets to the algebraic/topological properties of the Stone-Čech compactification of
$\mathop{({x}_{n} )}\nolimits_{n\in \mathbb{N} } $ of natural numbers. Central sets, first introduced by Furstenberg using notions from topological dynamics, constitute a special class of IP-sets possessing rich combinatorial properties: each central set contains arbitrarily long arithmetic progressions and solutions to all partition regular systems of homogeneous linear equations. In this paper we investigate central sets in the framework of combinatorics on words. Using various families of uniformly recurrent words, including Sturmian words, the Thue–Morse word and fixed points of weak mixing substitutions, we generate an assortment of central sets which reflect the rich combinatorial structure of the underlying words. The results in this paper rely on interactions between different areas of mathematics, some of which have not previously been directly linked. They include the general theory of combinatorics on words, abstract numeration systems, and the beautiful theory, developed by Hindman, Strauss and others, linking IP-sets and central sets to the algebraic/topological properties of the Stone-Čech compactification of 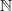 $ \mathbb{N} $.
$ \mathbb{N} $.
Information
- Type
- Research Article
- Information
- Copyright
- © Cambridge University Press, 2013
References
- 2
- Cited by

