Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Pan, Wenyu
2017.
Effective equidistribution of circles in the limit sets of Kleinian groups.
Journal of Modern Dynamics,
Vol. 11,
Issue. 1,
p.
189.
Parkkonen, Jouni
and
Paulin, Frédéric
2017.
Ergodic Theory and Negative Curvature.
Vol. 2164,
Issue. ,
p.
293.
Parkkonen, Jouni
and
Paulin, Frédéric
2022.
Rigidity, counting and equidistribution of quaternionic Cartan chains.
Annales Mathématiques Blaise Pascal,
Vol. 28,
Issue. 1,
p.
45.
Parkkonen, Jouni
and
Paulin, Frédéric
2022.
On the statistics of pairs of logarithms of integers.
Moscow Journal of Combinatorics and Number Theory,
Vol. 11,
Issue. 4,
p.
335.
PARKKONEN, JOUNI
and
PAULIN, FRÉDÉRIC
2022.
Counting and equidistribution in quaternionic Heisenberg groups.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 173,
Issue. 1,
p.
67.
Liu, Beibei
and
Vargas Pallete, Franco
2023.
Uniform spectral gap and orthogeodesic counting for strong convergence of Kleinian groups.
Forum of Mathematics, Sigma,
Vol. 11,
Issue. ,
Carvajales, León
2023.
Growth of Quadratic Forms Under Anosov Subgroups.
International Mathematics Research Notices,
Vol. 2023,
Issue. 1,
p.
785.
Dang, Nguyen Viet
and
Rivière, Gabriel
2024.
Poincaré series and linking of Legendrian knots.
Duke Mathematical Journal,
Vol. 173,
Issue. 1,
PARKKONEN, JOUNI
and
PAULIN, FRÉDÉRIC
2024.
Joint partial equidistribution of Farey rays in negatively curved manifolds and trees.
Ergodic Theory and Dynamical Systems,
Vol. 44,
Issue. 9,
p.
2700.
Edwards, Sam
Lee, Minju
and
Oh, Hee
2024.
Torus counting and self-joinings of Kleinian groups.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 0,
Issue. 0,
Parkkonen, Jouni
and
Paulin, Frédéric
2024.
Pair correlations of logarithms of complex lattice points.
Research in Number Theory,
Vol. 10,
Issue. 2,
Han, Suzhen
Yang, Wenyuan
and
Zou, Yanqing
2025.
Counting double cosets with application to generic 3‐manifolds.
Journal of Topology,
Vol. 18,
Issue. 3,
Basmajian, Ara
Parlier, Hugo
and
Tan, Ser Peow
2025.
Prime orthogeodesics, concave cores and families of identities on hyperbolic surfaces.
Advances in Mathematics,
Vol. 460,
Issue. ,
p.
110026.
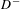 $D^{-}$ and
$D^{-}$ and 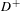 $D^{+}$ be properly immersed closed locally convex subsets of a Riemannian manifold with pinched negative sectional curvature. Using mixing properties of the geodesic flow, we give an asymptotic formula as
$D^{+}$ be properly immersed closed locally convex subsets of a Riemannian manifold with pinched negative sectional curvature. Using mixing properties of the geodesic flow, we give an asymptotic formula as 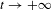 $t\rightarrow +\infty$ for the number of common perpendiculars of length at most
$t\rightarrow +\infty$ for the number of common perpendiculars of length at most  $t$ from
$t$ from 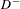 $D^{-}$ to
$D^{-}$ to 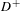 $D^{+}$ , counted with multiplicities, and we prove the equidistribution in the outer and inner unit normal bundles of
$D^{+}$ , counted with multiplicities, and we prove the equidistribution in the outer and inner unit normal bundles of 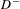 $D^{-}$ and
$D^{-}$ and 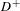 $D^{+}$ of the tangent vectors at the endpoints of the common perpendiculars. When the manifold is compact with exponential decay of correlations or arithmetic with finite volume, we give an error term for the asymptotic. As an application, we give an asymptotic formula for the number of connected components of the domain of discontinuity of Kleinian groups as their diameter goes to
$D^{+}$ of the tangent vectors at the endpoints of the common perpendiculars. When the manifold is compact with exponential decay of correlations or arithmetic with finite volume, we give an error term for the asymptotic. As an application, we give an asymptotic formula for the number of connected components of the domain of discontinuity of Kleinian groups as their diameter goes to  $0$ .
$0$ .
