Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Denzler, Jochen
1987.
Mather sets for plane Hamiltonian systems.
ZAMP Zeitschrift f�r angewandte Mathematik und Physik,
Vol. 38,
Issue. 6,
p.
791.
Mather, John N.
1989.
Minimal measures.
Commentarii Mathematici Helvetici,
Vol. 64,
Issue. 1,
p.
375.
Moser, Jürgen
1989.
Quasi-periodic solutions of nonlinear elliptic partial differential equations.
Boletim da Sociedade Brasileira de Matemática,
Vol. 20,
Issue. 1,
p.
29.
Haydn, N T A
1990.
On invariant curves under renormalisation.
Nonlinearity,
Vol. 3,
Issue. 3,
p.
887.
Mather, John N.
1990.
Differentiability of the minimal average action as a function of the rotation number.
Boletim da Sociedade Brasileira de Matem�tica,
Vol. 21,
Issue. 1,
p.
59.
Bangert, Victor
1990.
Minimal geodesics.
Ergodic Theory and Dynamical Systems,
Vol. 10,
Issue. 2,
p.
263.
Bangert, V.
1990.
Analysis, et Cetera.
p.
85.
Mather, John N.
1991.
Variational construction of orbits of twist diffeomorphisms.
Journal of the American Mathematical Society,
Vol. 4,
Issue. 2,
p.
207.
MacKay, R S
and
Stark, J
1992.
Locally most robust circles and boundary circles for area-preserving maps.
Nonlinearity,
Vol. 5,
Issue. 4,
p.
867.
Falcolini, Corrado
and
de la Llave, Rafael
1992.
A rigorous partial justification of Greene's criterion.
Journal of Statistical Physics,
Vol. 67,
Issue. 3-4,
p.
609.
Mather, John N.
and
Forni, Giovanni
1994.
Transition to Chaos in Classical and Quantum Mechanics.
Vol. 1589,
Issue. ,
p.
92.
Forni, Giovanni
1994.
Analytic destruction of invariant circles.
Ergodic Theory and Dynamical Systems,
Vol. 14,
Issue. 2,
p.
267.
Levi, M.
and
Zehnder, E.
1995.
Boundedness of Solutions for Quasiperiodic Potentials.
SIAM Journal on Mathematical Analysis,
Vol. 26,
Issue. 5,
p.
1233.
Li, Baowen
and
Robnik, M
1995.
Geometry of high-lying eigenfunctions in a plane billiard system having mixed-type classical dynamics.
Journal of Physics A: Mathematical and General,
Vol. 28,
Issue. 10,
p.
2799.
Huang, Xianhua
1996.
Nonlinear Dynamical Systems and Chaos.
p.
373.
Forni, Giovanni
1996.
Construction of invariant measures supported within the gaps of Aubry–Mather sets.
Ergodic Theory and Dynamical Systems,
Vol. 16,
Issue. 1,
p.
51.
Huang, Hai
1998.
Destruction of Invariant Tori in Pendulum-Type Equations.
Journal of Differential Equations,
Vol. 146,
Issue. 1,
p.
67.
Huang, Hai
1998.
Generic Existence of Invariant Cantori in Pendulum-Type Equations.
Journal of Mathematical Analysis and Applications,
Vol. 218,
Issue. 2,
p.
379.
Ruggiero, Rafael
2000.
On the generic nonexistence of rational geodesic foliations in the torus, Mather sets and Gromov hyperbolic spaces.
Boletim da Sociedade Brasileira de Matem�tica,
Vol. 31,
Issue. 1,
p.
93.
Shang, Zai-jiu
2000.
Resonant and Diophantine step sizes in computing invariant tori of Hamiltonian systems.
Nonlinearity,
Vol. 13,
Issue. 1,
p.
299.

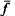 in be an exact, area-preserving, monotone twist diffeomorphism of the cylinder and let 10 be a Liouville number. We will show that arbitrarily close to
in be an exact, area-preserving, monotone twist diffeomorphism of the cylinder and let 10 be a Liouville number. We will show that arbitrarily close to 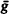 with no homotopically non-trivial invariant circle of rotation number ω.
with no homotopically non-trivial invariant circle of rotation number ω.