Article contents
Effective mixing and counting inBruhat–Tits trees
Published online by Cambridge University Press: 04 July 2016
Abstract
Let 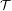 ${\mathcal{T}}$ be a locally finite tree,
${\mathcal{T}}$ be a locally finite tree,  $\unicode[STIX]{x1D6E4}$ be a discrete subgroup of
$\unicode[STIX]{x1D6E4}$ be a discrete subgroup of  $\,\operatorname{Aut}\,({\mathcal{T}})$ and
$\,\operatorname{Aut}\,({\mathcal{T}})$ and 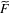 $\widetilde{F}$ be a
$\widetilde{F}$ be a 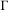 $\unicode[STIX]{x1D6E4}$ -invariant potential. Suppose that the length spectrum of
$\unicode[STIX]{x1D6E4}$ -invariant potential. Suppose that the length spectrum of 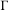 $\unicode[STIX]{x1D6E4}$ is not arithmetic. In this case, we prove the exponential mixing property of the geodesic translation map
$\unicode[STIX]{x1D6E4}$ is not arithmetic. In this case, we prove the exponential mixing property of the geodesic translation map  $\unicode[STIX]{x1D719}:\unicode[STIX]{x1D6E4}\backslash S{\mathcal{T}}\rightarrow \unicode[STIX]{x1D6E4}\backslash S{\mathcal{T}}$ with respect to the measure
$\unicode[STIX]{x1D719}:\unicode[STIX]{x1D6E4}\backslash S{\mathcal{T}}\rightarrow \unicode[STIX]{x1D6E4}\backslash S{\mathcal{T}}$ with respect to the measure 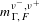 $m_{\unicode[STIX]{x1D6E4},F}^{\unicode[STIX]{x1D708}^{-},\unicode[STIX]{x1D708}^{+}}$ under the assumption that
$m_{\unicode[STIX]{x1D6E4},F}^{\unicode[STIX]{x1D708}^{-},\unicode[STIX]{x1D708}^{+}}$ under the assumption that 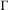 $\unicode[STIX]{x1D6E4}$ is full and
$\unicode[STIX]{x1D6E4}$ is full and 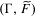 $(\unicode[STIX]{x1D6E4},\widetilde{F})$ has a weighted spectral gap. We also obtain the effective formula for the number of
$(\unicode[STIX]{x1D6E4},\widetilde{F})$ has a weighted spectral gap. We also obtain the effective formula for the number of 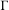 $\unicode[STIX]{x1D6E4}$ -orbits with weights in a Bruhat–Tits tree
$\unicode[STIX]{x1D6E4}$ -orbits with weights in a Bruhat–Tits tree 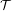 ${\mathcal{T}}$ of an algebraic group.
${\mathcal{T}}$ of an algebraic group.
Information
- Type
- Research Article
- Information
- Copyright
- © Cambridge University Press, 2016
References
A correction has been issued for this article:
- 3
- Cited by
Linked content
Please note a has been issued for this article.

