Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-11T03:49:42.993Z Has data issue: false hasContentIssue false
  • English
  • Français

Entropy of general diffeomorphisms on line

Part of: Topological dynamics Low-dimensional dynamical systems

Published online by Cambridge University Press:  18 February 2020

BAOLIN HE
BAOLIN HE*
Affiliation:
Shanghai Normal University, Mathematics, Shanghai, 200234, PR China email hebaolin@shnu.edu.cn
Get access Rights & Permissions [Opens in a new window]

Abstract

We study the continuity of topological entropy of general diffeomorphisms on line. First, we prove that the entropy map is continuous with respect to the strong 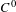$C^{0}$-topology on the union of uniformly topologically hyperbolic diffeomorphisms contained in 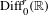$\text{Diff}_{0}^{r}(\mathbb{R})$ (whose first derivative is uniformly away from zero), which is a 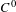$C^{0}$-open and 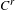$C^{r}$-dense subset of 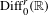$\text{Diff}_{0}^{r}(\mathbb{R})$, 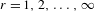$r=1,2,\ldots ,\infty$, and $\unicode[STIX]{x1D714}$ (real analytic). Second, we give some examples where entropy map is not continuous. Finally, we prove some results on the continuity of entropy of general diffeomorphisms on the (real) line.

Keywords

dimension theorysmooth dynamicstopological dynamics

MSC classification

Primary: 37B40: Topological entropy 37E05: Maps of the interval (piecewise continuous, continuous, smooth)
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 41 , Issue 4 , April 2021 , pp. 1139 - 1155
Check for updates
Copyright
© The Author(s) 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

He, B.. Entropy of diffeomorphisms of line. Discrete Contin. Dyn. Syst. 37 (2017), 4753–4766.10.3934/dcds.2017204CrossRefGoogle Scholar
Liao, G., Viana, M. and Yang, J.. The entropy conjecture for diffeomorphisms away from tangencies. J. Eur. Math. Soc. (JEMS) 15 (2013), 2043–2060.10.4171/JEMS/413CrossRefGoogle Scholar
Milnor, J. and Thurston, W.. On iterated maps of the interval. Dynamical Systems (Lecture Notes in Mathematics, 1342) . Springer, Berlin, 1988, pp. 465–563.10.1007/BFb0082847CrossRefGoogle Scholar
Newhouse, S.. Continuity properties of entropy. Ann. of Math. 129 (1989), 215–235.10.2307/1971492CrossRefGoogle Scholar
Saghin, R. and Yang, J.. Continuity of topological entropy for perturbation of time-one maps of hyperbolic flows. Israel J. Math. 215 (2016), 857–875.10.1007/s11856-016-1396-4CrossRefGoogle Scholar
Walters, P.. Ergodic Theory: Introductory Lectures (Lecture Notes in Mathematics, 458) . Springer, Berlin, 1975.Google Scholar
Yomdin, Y.. Volume growth and entropy. Israel J. Math. 57 (1987), 285–300.Google Scholar