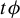Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Barbieri, Sebastián
Bissacot, Rodrigo
Dalle Vedove, Gregório
and
Thieullen, Philippe
2024.
Zero-temperature chaos in bidimensional models with finite-range potentials.
Advances in Mathematics,
Vol. 457,
Issue. ,
p.
109906.