Article contents
Four-cycle free graphs, height functions, the pivot property and entropy minimality
Published online by Cambridge University Press: 08 March 2016
Abstract
Fix  $d\geq 2$ . Given a finite undirected graph
$d\geq 2$ . Given a finite undirected graph 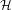 ${\mathcal{H}}$ without self-loops and multiple edges, consider the corresponding ‘vertex’ shift,
${\mathcal{H}}$ without self-loops and multiple edges, consider the corresponding ‘vertex’ shift,  $\text{Hom}(\mathbb{Z}^{d},{\mathcal{H}})$ , denoted by
$\text{Hom}(\mathbb{Z}^{d},{\mathcal{H}})$ , denoted by 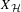 $X_{{\mathcal{H}}}$ . In this paper, we focus on
$X_{{\mathcal{H}}}$ . In this paper, we focus on 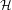 ${\mathcal{H}}$ which is ‘four-cycle free’. There are two main results of this paper. Firstly, that
${\mathcal{H}}$ which is ‘four-cycle free’. There are two main results of this paper. Firstly, that 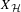 $X_{{\mathcal{H}}}$ has the pivot property, meaning that, for all distinct configurations
$X_{{\mathcal{H}}}$ has the pivot property, meaning that, for all distinct configurations 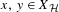 $x,y\in X_{{\mathcal{H}}}$ , which differ only at a finite number of sites, there is a sequence of configurations
$x,y\in X_{{\mathcal{H}}}$ , which differ only at a finite number of sites, there is a sequence of configurations 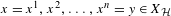 $x=x^{1},x^{2},\ldots ,x^{n}=y\in X_{{\mathcal{H}}}$ for which the successive configurations
$x=x^{1},x^{2},\ldots ,x^{n}=y\in X_{{\mathcal{H}}}$ for which the successive configurations  $x^{i},x^{i+1}$ differ exactly at a single site. Secondly, if
$x^{i},x^{i+1}$ differ exactly at a single site. Secondly, if 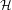 ${\mathcal{H}}$ is connected ,then
${\mathcal{H}}$ is connected ,then 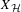 $X_{{\mathcal{H}}}$ is entropy minimal, meaning that every shift space strictly contained in
$X_{{\mathcal{H}}}$ is entropy minimal, meaning that every shift space strictly contained in  $X_{{\mathcal{H}}}$ has strictly smaller entropy. The proofs of these seemingly disparate statements are related by the use of the ‘lifts’ of the configurations in
$X_{{\mathcal{H}}}$ has strictly smaller entropy. The proofs of these seemingly disparate statements are related by the use of the ‘lifts’ of the configurations in 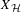 $X_{{\mathcal{H}}}$ to the universal cover of
$X_{{\mathcal{H}}}$ to the universal cover of 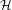 ${\mathcal{H}}$ and the introduction of ‘height functions’ in this context.
${\mathcal{H}}$ and the introduction of ‘height functions’ in this context.
Information
- Type
- Research Article
- Information
- Copyright
- © Cambridge University Press, 2016
References
- 2
- Cited by

