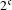A metric minimal PI cascade with $2^{\mathfrak{c}}$ minimal ideals

Abstract

We first improve an old result of McMahon and show that a metric minimal flow whose enveloping semigroup contains less than $2^{\mathfrak{c}}$ (where $\mathfrak{c}=2^{\aleph _{0}}$) minimal left ideals is proximal isometric (PI). Then we show the existence of various minimal PI-flows with many minimal left ideals, as follows. For the acting group 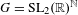$G=\text{SL}_{2}(\mathbb{R})^{\mathbb{N}}$, we construct a metric minimal PI 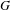$G$-flow with $\mathfrak{c}$ minimal left ideals. We then use this example and results established in Glasner and Weiss. [On the construction of minimal skew-products. Israel J. Math.34 (1979), 321–336] to construct a metric minimal PI cascade $(X,T)$ with $\mathfrak{c}$ minimal left ideals. We go on to construct an example of a minimal PI-flow 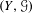$(Y,{\mathcal{G}})$ on a compact manifold $Y$ and a suitable path-wise connected group 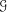${\mathcal{G}}$ of a homeomorphism of $Y$, such that the flow 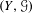$(Y,{\mathcal{G}})$ is PI and has 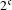$2^{\mathfrak{c}}$ minimal left ideals. Finally, we use this latter example and a theorem of Dirbák to construct a cascade 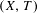$(X,T)$ that is PI (of order three) and has 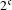$2^{\mathfrak{c}}$ minimal left ideals. Thus this final result shows that, even for cascades, the converse of the implication ‘less than 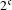$2^{\mathfrak{c}}$ minimal left ideals implies PI’, fails.