Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-11T07:14:57.686Z Has data issue: false hasContentIssue false
  • English
  • Français

On arithmetic progressions in non-periodic self-affine tilings

Part of: Discrete geometry

Published online by Cambridge University Press:  15 June 2021

YASUSHI NAGAI ,
SHIGEKI AKIYAMA  and
JEONG-YUP LEE
YASUSHI NAGAI*
Affiliation:
School of General Education, Shinshu University, 3-1-1 Asahi, Matsumoto, Nagano390-8621, Japan
SHIGEKI AKIYAMA
Affiliation:
Institute of Mathematics, University of Tsukuba, 1-1-1 Tennodai, Tsukuba, Ibaraki305-8571, Japan (e-mail: akiyama@math.tsukuba.ac.jp)
JEONG-YUP LEE
Affiliation:
Department of Mathematics Education, Catholic Kwandong University, Gangneung, Gangwon 210-701, Korea, or KIAS, 85 Hoegiro, Dongdaemun-gu, Seoul02455, Korea (e-mail: jylee@cku.ac.kr)
*
e-mail: ynagai@shinshu-u.ac.jp
Get access Rights & Permissions [Opens in a new window]

Abstract

We study the repetition of patches in self-affine tilings in 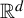${\mathbb {R}}^d$ . In particular, we study the existence and non-existence of arithmetic progressions. We first show that an arithmetic condition of the expansion map for a self-affine tiling implies the non-existence of certain one-dimensional arithmetic progressions. Next, we show that the existence of full-rank infinite arithmetic progressions, pure discrete dynamical spectrum, and limit-periodicity are all equivalent for a certain class of self-affine tilings. We finish by giving a complete picture for the existence or non-existence of full-rank infinite arithmetic progressions in the self-similar tilings in 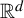${\mathbb {R}}^d$ .

Keywords

tilingtiling dynamical systemdynamical spectrumarithmetic progression

MSC classification

Secondary: 52C23: Quasicrystals, aperiodic tilings
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 42 , Issue 9 , September 2022 , pp. 2957 - 2989
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Akiyama, S. and Lee, J.-Y.. Algorithm for determining pure pointedness of self-affine tilings. Adv. Math. 226(4) (2011), 2855–2883.CrossRefGoogle Scholar
Akiyama, S. and Lee, J.-Y.. Overlap coincidence to strong coincidence in substitution tiling dynamics. Eur. J. Combin. 39 (2014), 233–243.CrossRefGoogle Scholar
Baake, M. and Grimm, U.. Diffraction of limit periodic point sets. Philos. Mag. 91(19–21) (2011), 2661–2670.CrossRefGoogle Scholar
Baake, M. and Grimm, U.. Aperiodic Order. Vol. 1: A Mathematical Invitation. Cambridge University Press, Cambridge, 2013.Google Scholar
Baake, M. and Lenz, D.. Dynamical systems on translation bounded measures: pure point dynamical and diffraction spectra. Ergod. Th. & Dynam. Sys. 24(6) (2004), 1867–1893.CrossRefGoogle Scholar
Baake, M., Moody, R. V. and Schlottmann, M.. Limit-(quasi) periodic point sets as quasicrystals with p-adic internal spaces. J. Phys. A: Math. Gen. 31(27) (1998), 5755.CrossRefGoogle Scholar
de la Llave, R. and Windsor, A.. An application of topological multiple recurrence to tiling. Discrete Contin. Dyn. Syst. 2(2) (2009), 315–324.Google Scholar
Frettlöh, D.. Nichtperiodische Pflasterungen mit ganzzahligem Inflationsfaktor. PhD Thesis, Universität Dortmund, 2002.Google Scholar
Furstenberg, H.. Recurrence in Ergodic Theory and Combinatorial Number Theory. Princeton University Press, Princeton, NJ, 2014.Google Scholar
Gähler, F. and Klitzing, R.. The diffraction pattern of self-similar tilings. NATO ASI C Math. Phys. Sci. Adv. Study Inst. 489 (1997), 141–174.Google Scholar
Godreche, C.. The sphinx: a limit-periodic tiling of the plane. J. Phys. A: Math. Gen. 22(24) (1989), L1163.CrossRefGoogle Scholar
Gouéré, J-B.. Quasicrystals and almost periodicity. Commun. Math. Phys. 255(3) (2005), 655–681.CrossRefGoogle Scholar
Iwanik, A. and Lacroix, Y.. Some constructions of strictly ergodic non-regular Toeplitz flows. Studia Math. 110(2) (1994), 191–203.Google Scholar
Jacobs, K. and Keane, M.. 0-1-sequences of Toeplitz type. Z. Wahrsch. Verw. Geb. 13(2) (1969), 123–131.CrossRefGoogle Scholar
Klick, A., Strungaru, N. and Tcaciuc, A.. On arithmetic progressions in model sets. Preprint, 2021, arXiv:2003.13860.CrossRefGoogle Scholar
Lee, J.-Y.. Substitution Delone sets with pure point spectrum are inter-model sets. J. Geom. Phys. 57(11) (2007), 2263–2285.CrossRefGoogle Scholar
Lee, J.-Y., Moody, R. V. and Solomyak, B.. Consequences of pure point diffraction spectra for multiset substitution systems. Discrete Comput. Geom. 29(4) (2003), 525–560.CrossRefGoogle Scholar
Lee, J.-Y. and Solomyak, B.. Pure point diffractive substitution Delone sets have the Meyer property. Discr. Comput. Geom. 39 (2008), 319–338.CrossRefGoogle Scholar
Lee, J.-Y. and Solomyak, B.. Pisot family substitution tilings, discrete spectrum and the Meyer property. Discrete Contin. Dyn. Syst. 32(3) (2012), 935–959.CrossRefGoogle Scholar
Lenz, D., Spindeler, T. and Strungaru, N.. Pure point diffraction and mean, Besicovitch and Weyl almost periodicity. Preprint, 2021, arXiv:2006.10821 Google Scholar
Mañibo, N.. Lyapunov exponents in the spectral theory of primitive inflation systems. PhD Thesis, Universität Bielefeld, 2019.Google Scholar
Nagai, Y.. The common structure for objects in aperiodic order and the theory of local matching topology. Preprint, 2021, arXiv:1811.04642 Google Scholar
Praggastis, B.. Markov partitions for hyperbolic toral automorphisms. PhD Thesis, University of Washington, 1992.Google Scholar
Praggastis, B.. Numeration systems and Markov partitions from self similar tilings. Trans. Amer. Math. Soc. 351(8) (1999), 3315–3349.CrossRefGoogle Scholar
Queffélec, M.. Substitution Dynamical Systems—Spectral Analysis (Lecture Notes in Mathematics, 1294). Springer, New York, 2010.CrossRefGoogle Scholar
Solomyak, B.. Dynamics of self-similar tilings. Ergod. Th. & Dynam. Sys. 17(3) (1997), 695–738.CrossRefGoogle Scholar
Solomyak, B.. Eigenfunctions for substitution tiling systems. Probability and Number Theory, Kanazawa, 2005 (Advanced Studies in Pure Mathematics, 49). Mathematical Society of Japan, Tokyo, 2007, pp. 433–454.CrossRefGoogle Scholar