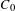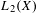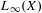Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Arendt, W.
and
ter Elst, A. F. M.
2017.
Ultracontractivity and Eigenvalues: Weyl’s Law for the Dirichlet-to-Neumann Operator.
Integral Equations and Operator Theory,
Vol. 88,
Issue. 1,
p.
65.
Edeko, Nikolai
Gerlach, Moritz
and
Kühner, Viktoria
2019.
Measure-preserving semiflows and one-parameter Koopman semigroups.
Semigroup Forum,
Vol. 98,
Issue. 1,
p.
48.
Giannakis, Dimitrios
2019.
Data-driven spectral decomposition and forecasting of ergodic dynamical systems.
Applied and Computational Harmonic Analysis,
Vol. 47,
Issue. 2,
p.
338.
Grotto, Francesco
2020.
Essential self-adjointness of Liouville operator for 2D Euler point vortices.
Journal of Functional Analysis,
Vol. 279,
Issue. 6,
p.
108635.
Kandić, M.
and
Kaplin, M.
2020.
Relatively uniformly continuous semigroups on vector lattices.
Journal of Mathematical Analysis and Applications,
Vol. 489,
Issue. 1,
p.
124139.
Giannakis, Dimitrios
2021.
Quantum dynamics of the classical harmonic oscillator.
Journal of Mathematical Physics,
Vol. 62,
Issue. 4,
Giannakis, Dimitrios
Ourmazd, Abbas
Pfeffer, Philipp
Schumacher, Jörg
and
Slawinska, Joanna
2022.
Embedding classical dynamics in a quantum computer.
Physical Review A,
Vol. 105,
Issue. 5,