Article contents
Random local complex dynamics
Published online by Cambridge University Press: 26 December 2018
Abstract
The study of the dynamics of an holomorphic map near a fixed point is a central topic in complex dynamical systems. In this paper, we will consider the corresponding random setting: given a probability measure  $\unicode[STIX]{x1D708}$ with compact support on the space of germs of holomorphic maps fixing the origin, we study the compositions
$\unicode[STIX]{x1D708}$ with compact support on the space of germs of holomorphic maps fixing the origin, we study the compositions  $f_{n}\circ \cdots \circ f_{1}$, where each
$f_{n}\circ \cdots \circ f_{1}$, where each 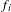 $f_{i}$ is chosen independently with probability
$f_{i}$ is chosen independently with probability  $\unicode[STIX]{x1D708}$. As in the deterministic case, the stability of the family of the random iterates is mostly determined by the linear part of the germs in the support of the measure. A particularly interesting case occurs when all Lyapunov exponents vanish, in which case stability implies simultaneous linearizability of all germs in
$\unicode[STIX]{x1D708}$. As in the deterministic case, the stability of the family of the random iterates is mostly determined by the linear part of the germs in the support of the measure. A particularly interesting case occurs when all Lyapunov exponents vanish, in which case stability implies simultaneous linearizability of all germs in 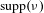 $\text{supp}(\unicode[STIX]{x1D708})$.
$\text{supp}(\unicode[STIX]{x1D708})$.
MSC classification
Information
- Type
- Original Article
- Information
- Copyright
- © Cambridge University Press, 2018
References
- 1
- Cited by


