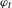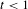Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Climenhaga, Vaughn
and
Thompson, Daniel J.
2021.
Thermodynamic Formalism.
Vol. 2290,
Issue. ,
p.
3.
HASSELBLATT, BORIS
2022.
Anatole Katok.
Ergodic Theory and Dynamical Systems,
Vol. 42,
Issue. 2,
p.
321.
CALL, BENJAMIN
2022.
The K-property for some unique equilibrium states in flows and homeomorphisms.
Ergodic Theory and Dynamical Systems,
Vol. 42,
Issue. 8,
p.
2509.
Hou, Xiaobo
Tian, Xueting
and
Zhao, Xutong
2025.
Chaoticity of generic points for ergodic measures in hyperbolic systems and beyond.
Journal of Differential Equations,
Vol. 436,
Issue. ,
p.
113236.
Dong, Changguang
and
Qiao, Qiujie
2025.
On r-Neutralized Entropy: Entropy Formula and Existence of Measures Attaining the Supremum.
Communications in Mathematical Physics,
Vol. 406,
Issue. 4,