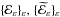Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Du, Qiang
and
Feng, Xiaobing
2020.
Geometric Partial Differential Equations - Part I.
Vol. 21,
Issue. ,
p.
425.
Dabrock, Nils
Knüttel, Sascha
and
Röger, Matthias
2023.
“Gradient-free” diffuse approximations of the Willmore functional and Willmore flow.
Asymptotic Analysis,
Vol. 133,
Issue. 1-2,
p.
185.
Nguyen, Huy The
and
Wang, Shengwen
2024.
Quantization of the energy for the inhomogeneous Allen–Cahn mean curvature.
Mathematische Annalen,
Vol. 390,
Issue. 4,
p.
6399.