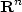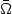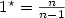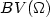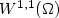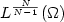Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Demengel, Françoise
2004.
Functions locally almost 1-harmonic.
Applicable Analysis,
Vol. 83,
Issue. 9,
p.
865.
Saintier, Nicolas
2008.
Estimates of the best Sobolev constant of the embedding of into and related shape optimization problems.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 69,
Issue. 8,
p.
2479.
Kraïem, Mouna
2008.
On some nonlinear partial differential equations involving the 1-Laplacian.
Annales de la Faculté des sciences de Toulouse : Mathématiques,
Vol. 16,
Issue. 4,
p.
905.
CHANG, KUNG CHING
2009.
THE SPECTRUM OF THE 1-LAPLACE OPERATOR.
Communications in Contemporary Mathematics,
Vol. 11,
Issue. 05,
p.
865.
Degiovanni, Marco
and
Magrone, Paola
2009.
Linking solutions for quasilinear equations at critical growth involving the “1-Laplace” operator.
Calculus of Variations and Partial Differential Equations,
Vol. 36,
Issue. 4,
p.
591.
Bartsch, Thomas
and
Willem, Michel
2010.
Some critical minimization problems for functions of bounded variations.
Journal of Functional Analysis,
Vol. 259,
Issue. 11,
p.
3025.
Duchateau, Xavier
2013.
On some quasilinear equations involving the p-Laplacian with Robin boundary conditions.
Applicable Analysis,
Vol. 92,
Issue. 2,
p.
270.
Amendola, Maria Emilia
Gargiulo, Giuliano
and
Zappale, Elvira
2014.
Dimension reduction for −Δ1.
ESAIM: Control, Optimisation and Calculus of Variations,
Vol. 20,
Issue. 1,
p.
42.
Scheven, Christoph
and
Schmidt, Thomas
2016.
BV supersolutions to equations of 1-Laplace and minimal surface type.
Journal of Differential Equations,
Vol. 261,
Issue. 3,
p.
1904.
Scheven, Christoph
and
Schmidt, Thomas
2018.
On the dual formulation of obstacle problems for the total variation and the area functional.
Annales de l'Institut Henri Poincaré C, Analyse non linéaire,
Vol. 35,
Issue. 5,
p.
1175.
Figueiredo, Giovany M.
and
Pimenta, Marcos T.O.
2018.
Existence of bounded variation solutions for a 1-Laplacian problem with vanishing potentials.
Journal of Mathematical Analysis and Applications,
Vol. 459,
Issue. 2,
p.
861.
Molino Salas, Alexis
and
Segura de León, Sergio
2018.
Elliptic equations involving the 1-Laplacian and a subcritical source term.
Nonlinear Analysis,
Vol. 168,
Issue. ,
p.
50.
Figueiredo, Giovany M.
and
Pimenta, Marcos T. O.
2018.
Strauss’ and Lions’ Type Results in
$${BV (\mathbb{R}^N}$$
B
V
(
R
N
) with an Application to an 1-Laplacian Problem.
Milan Journal of Mathematics,
Vol. 86,
Issue. 1,
p.
15.
De Cicco, Virginia
Giachetti, Daniela
Oliva, Francescantonio
and
Petitta, Francesco
2019.
The Dirichlet problem for singular elliptic equations with general nonlinearities.
Calculus of Variations and Partial Differential Equations,
Vol. 58,
Issue. 4,
Dall’Aglio, A.
and
Segura de León, S.
2019.
Bounded solutions to the 1-Laplacian equation with a total variation term.
Ricerche di Matematica,
Vol. 68,
Issue. 2,
p.
597.
Oliva, Francescantonio
2019.
Regularizing effect of absorption terms in singular problems.
Journal of Mathematical Analysis and Applications,
Vol. 472,
Issue. 1,
p.
1136.
de la Calle Ysern, B.
Sabina de Lis, J.C.
and
Segura de León, S.
2020.
The convective eigenvalues of the one–dimensional p–Laplacian as p → 1.
Journal of Mathematical Analysis and Applications,
Vol. 484,
Issue. 1,
p.
123738.
Alves, Claudianor O.
Figueiredo, Giovany M.
and
Pimenta, Marcos T. O.
2020.
Existence and Profile of Ground-State Solutions to a 1-Laplacian Problem in $$\mathbb {R}^N$$.
Bulletin of the Brazilian Mathematical Society, New Series,
Vol. 51,
Issue. 3,
p.
863.
Wei, Yawei
and
Zhao, Huiying
2020.
Dirichlet problems involving the 1-Laplacian.
Journal of Pseudo-Differential Operators and Applications,
Vol. 11,
Issue. 4,
p.
1897.
Latorre, Marta
Oliva, Francescantonio
Petitta, Francesco
and
Segura de León, Sergio
2021.
The Dirichlet problem for the 1-Laplacian with a general singular term and L
1-data.
Nonlinearity,
Vol. 34,
Issue. 3,
p.
1791.