Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Sander, Evelyn
and
Wanner, Thomas
2000.
Unexpectedly Linear Behavior for the Cahn--Hilliard Equation.
SIAM Journal on Applied Mathematics,
Vol. 60,
Issue. 6,
p.
2182.
Trabelsi, Karim
2003.
Nonlinear thin plate models for a family of Ogden materials.
Comptes Rendus. Mathématique,
Vol. 337,
Issue. 12,
p.
819.
Trabelsi, Karim
2004.
Incompressible nonlinearly elastic thin membranes.
Comptes Rendus. Mathématique,
Vol. 340,
Issue. 1,
p.
75.
TRABELSI, KARIM
2005.
NONLINEARLY ELASTIC THIN PLATE MODELS FOR A CLASS OF OGDEN MATERIALS: I.
Analysis and Applications,
Vol. 03,
Issue. 02,
p.
195.
Trabelsi, Karim
2006.
On Nonlinearly Elastic Membranes under Compression.
Chinese Annals of Mathematics, Series B,
Vol. 27,
Issue. 4,
p.
379.
TRABELSI, KARIM
2006.
MODELING OF A MEMBRANE FOR NONLINEARLY ELASTIC INCOMPRESSIBLE MATERIALS VIA GAMMA-CONVERGENCE.
Analysis and Applications,
Vol. 04,
Issue. 01,
p.
31.
Hafsa, Omar Anza
and
Mandallena, Jean-Philippe
2007.
Relaxation of variational problems in two-dimensional nonlinear elasticity.
Annali di Matematica Pura ed Applicata,
Vol. 186,
Issue. 1,
p.
185.
Anza Hafsa, Omar
and
Mandallena, Jean-Philippe
2008.
Relaxation theorems in nonlinear elasticity.
Annales de l'Institut Henri Poincaré C, Analyse non linéaire,
Vol. 25,
Issue. 1,
p.
135.
Freddi, Lorenzo
and
Paroni, Roberto
2008.
IUTAM Symposium on Relations of Shell Plate Beam and 3D Models.
Vol. 9,
Issue. ,
p.
111.
Yan, Baisheng
2009.
An explicit formula of reduced membrane energy for incompressiblep-Dirichlet energy.
Applicable Analysis,
Vol. 88,
Issue. 9,
p.
1321.
Anza Hafsa, Omar
2010.
On the integral representation of relaxed functionals with convex bounded constraints.
ESAIM: Control, Optimisation and Calculus of Variations,
Vol. 16,
Issue. 1,
p.
37.
Anza Hafsa, Omar
and
Mandallena, Jean-Philippe
2011.
Homogenization of nonconvex integrals with convex growth.
Journal de Mathématiques Pures et Appliquées,
Vol. 96,
Issue. 2,
p.
167.
Anza Hafsa, Omar
and
Mandallena, Jean-Philippe
2012.
Homogenization of unbounded singular integrals in W 1,∞.
Ricerche di Matematica,
Vol. 61,
Issue. 2,
p.
185.
Kružík, Martin
and
Zimmer, Johannes
2013.
Recent Trends in Dynamical Systems.
Vol. 35,
Issue. ,
p.
587.
Goldman, M.
and
Zwicknagl, B.
2014.
Scaling Law and Reduced Models for Epitaxially Strained Crystalline Films.
SIAM Journal on Mathematical Analysis,
Vol. 46,
Issue. 1,
p.
1.
Kristensen, Jan
2015.
A necessary and sufficient condition for lower semicontinuity.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 120,
Issue. ,
p.
43.
Conti, Sergio
and
Dolzmann, Georg
2015.
On the Theory of Relaxation in Nonlinear Elasticity with Constraints on the Determinant.
Archive for Rational Mechanics and Analysis,
Vol. 217,
Issue. 2,
p.
413.
Koumatos, Konstantinos
Rindler, Filip
and
Wiedemann, Emil
2016.
ORIENTATION-PRESERVING YOUNG MEASURES.
The Quarterly Journal of Mathematics,
Vol. 67,
Issue. 3,
p.
439.
Mohammadi, M.
Mohseni, E.
and
Moeinfar, M.
2019.
Bending, buckling and free vibration analysis of incompressible functionally graded plates using higher order shear and normal deformable plate theory.
Applied Mathematical Modelling,
Vol. 69,
Issue. ,
p.
47.
Barroso, Ana Cristina
and
Zappale, Elvira
2019.
Relaxation for Optimal Design Problems with Non-standard Growth.
Applied Mathematics & Optimization,
Vol. 80,
Issue. 2,
p.
515.
 matrices $\scriptstyle f: M^{m\times n}\rightarrow \bar{\mathbb{R}}$
matrices $\scriptstyle f: M^{m\times n}\rightarrow \bar{\mathbb{R}}$ 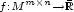 taking the value $ \scriptstyle +\infty$
taking the value $ \scriptstyle +\infty$ 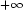 , such that its rank-one-convex envelope $\scriptstyle Rf$
, such that its rank-one-convex envelope $\scriptstyle Rf$ 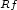 is finite and satisfies for some fixed $\scriptstyle p>1$
is finite and satisfies for some fixed $\scriptstyle p>1$ 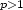 : $$\scriptstyle -c_0\leq Rf(F)\leq c(1+\Vert F\Vert^p)\ \hbox{for all}\F\in M^{m\times n},$$
: $$\scriptstyle -c_0\leq Rf(F)\leq c(1+\Vert F\Vert^p)\ \hbox{for all}\F\in M^{m\times n},$$ 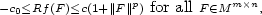 where $\scriptstyle c,c_0>0$
where $\scriptstyle c,c_0>0$  . Let $\scriptstyle\O$
. Let $\scriptstyle\O$  be a given regular bounded open domain of $\scriptstyle \mathbb{R}^n$
be a given regular bounded open domain of $\scriptstyle \mathbb{R}^n$ 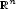 . We define on $\scriptstyle W^{1,p}(\O;\mathbb{R}^m)$
. We define on $\scriptstyle W^{1,p}(\O;\mathbb{R}^m)$ 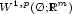 the functional $$\scriptstyle I(u)=\int_{\O}f(\nabla u(x))\ dx.$$
the functional $$\scriptstyle I(u)=\int_{\O}f(\nabla u(x))\ dx.$$ 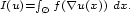 Then, under some technical restrictions on $\scriptstyle f$
Then, under some technical restrictions on $\scriptstyle f$ 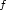 , we show that the relaxed functional $\scriptstyle\bar I$
, we show that the relaxed functional $\scriptstyle\bar I$ 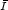 for the weak topology of $\scriptstyle W^{1,p}(\O;\mathbb{R}^m)$
for the weak topology of $\scriptstyle W^{1,p}(\O;\mathbb{R}^m)$ 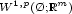 has the integral representation: $$\scriptstyle \bar I(u)=\int_{\O}Q[Rf](\nabla u(x))\ dx,$$
has the integral representation: $$\scriptstyle \bar I(u)=\int_{\O}Q[Rf](\nabla u(x))\ dx,$$  where for a given function $\scriptstyle g$
where for a given function $\scriptstyle g$  , $\scriptstyle Qg$
, $\scriptstyle Qg$ 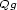 denotes its quasiconvex envelope.
denotes its quasiconvex envelope.