Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-27T15:10:57.822Z Has data issue: false hasContentIssue false
  • English
  • Français

Exponential deficiency of convolutions of densities∗

Published online by Cambridge University Press:  02 July 2012

Iosif Pinelis
Iosif Pinelis*
Affiliation:
Department of Mathematical Sciences, Michigan Technological University, Houghton, 49931 Michigan, USA. ipinelis@mtu.edu
Get access

Abstract

If a probability density p(x) (x ∈ ℝk) is bounded and R(t) := ∫e〈x, tu〉p(x)dx < ∞ for some linear functional u and all t ∈ (0,1), then, for each t ∈ (0,1) and all large enough n, the n-fold convolution of the t-tilted density \hbox{$\tilde p_t$}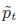˜pt := e〈x, tu〉p(x)/R(t) is bounded. This is a corollary of a general, “non-i.i.d.” result, which is also shown to enjoy a certain optimality property. Such results and their corollaries stated in terms of the absolute integrability of the corresponding characteristic functions are useful for saddle-point approximations.

Keywords

Probability densitysaddle-point approximationsums of independent random variables/vectorsconvolutionexponential integrabilityboundednessexponential tiltingexponential familiesabsolute integrabilitycharacteristic functions
Type
Research Article
Information
ESAIM: Probability and Statistics , Volume 16 , 2012 , pp. 86 - 96
Copyright
© EDP Sciences, SMAI, 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Références

Barndorff-Nielsen, O. and Cox, D.R., Edgeworth and saddle-point approximations with statistical applications. J. R. Stat. Soc., Ser. B 41 (1979) 279–312. With discussion. Google Scholar
R.N. Bhattacharya and R.R. Rao, Normal approximation and asymptotic expansions. Robert E. Krieger Publishing Co. Inc., Melbourne, FL (1986). Reprint of the 1976 original.
Daniels, H.E., Tail probability approximations. Int. Stat. Rev. 55 (1987) 37–48. Google Scholar
Embrechts, P. and Goldie, C.M., On convolution tails. Stoch. Proc. Appl. 13 (1982) 263–278. Google Scholar
Jing, B.-Y., Shao, Q.-M. and Zhou, W., Saddlepoint approximation for Student’s t-statistic with no moment conditions. Ann. Stat. 32 (2004) 2679–2711. Google Scholar
Klüppelberg, C., Subexponential distributions and characterizations of related classes. Probab. Theory Relat. Fields 82 (1989) 259–269. Google Scholar
Lugannani, R. and Rice, S., Saddle point approximation for the distribution of the sum of independent random variables. Adv. Appl. Probab. 12 (1980) 475–490. Google Scholar
Pinelis, I.F., Asymptotic equivalence of the probabilities of large deviations for sums and maximum of independent random variables, in Limit theorems of probability theory. “Nauka” Sibirsk. Otdel., Novosibirsk. Trudy Inst. Mat. 5 (1985) 144–173, 176. Google Scholar
Reid, N., Saddlepoint methods and statistical inference. Stat. Sci. 3 (1988) 213–238. With comments and a rejoinder by the author. Google Scholar
Q.-M. Shao, Recent progress on self-normalized limit theorems, in Probability, finance and insurance. World Sci. Publ., River Edge, NJ (2004) 50–68.