No CrossRef data available.
Article contents
Multiple Fourier analysis for bang-bang controls with memory
Published online by Cambridge University Press: 16 July 2009
Abstract
Bennett functions 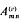 , (h, k), defined below and elsewhere, have been used extensively in Fourier analysing the output of certain devices such as rectifiers, limiters and other instantaneous modulators responding to a two-frequency input for which the output at time t depends only on the device input–output characteristic Y = Y(X) and the input at time t. Here, with the aid of a new set of modified Bennett functions
, (h, k), defined below and elsewhere, have been used extensively in Fourier analysing the output of certain devices such as rectifiers, limiters and other instantaneous modulators responding to a two-frequency input for which the output at time t depends only on the device input–output characteristic Y = Y(X) and the input at time t. Here, with the aid of a new set of modified Bennett functions  , (h, k), these methods are extended to solve the same problems for non-instantaneous bang-bang controls with multivalued input–output characteristics Y = Y(X) and hence having memory.
, (h, k), these methods are extended to solve the same problems for non-instantaneous bang-bang controls with multivalued input–output characteristics Y = Y(X) and hence having memory.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Cambridge University Press 1992
References
[1]Bennett, W. R. 1933 New results in the calculation of modulation products. Bell Syst. Tech. J. 12, 228–243.Google Scholar
[3]Sternberg, R. L. & Kaufman, H. 1953 A general solution of the two-frequency modulation product problem, I. J. Math. & Phys. 32, 233–242Google Scholar
[4]Sternberg, R. L. 1954 A general solution of the two frequency modulation product problem, II. Tables of the functions  (h, k). J. Math. & Phys. 33, 68–79.Google Scholar
(h, k). J. Math. & Phys. 33, 68–79.Google Scholar
[5]Sternberg, R. L. 1954 A general solution of the two frequency modulation product problem, IIIRectifiers and limiters. J. Math. & Phys. 33, 199–205.Google Scholar
[6]Sternberg, R. L., Shipman, J. S. & Thurston, W. B. 1954 Tables of Bennett functions for the two-frequency modulation product problem for the half-wave square-law rectifier. Quart. J. Mech. & Appl. Math. 7 (4), 505–509.Google Scholar
[7]Sternberg, R. L. & Shipman, J. S., Kaufman, H. 1955 Tables of Bennett functions for the two-frequency modulation product problem for the half-wave square-law rectifier. Quart. J. Mech. & Appl. Math. 8, 457–467.Google Scholar
[8]Sternberg, R. L., Shipman, J. S., Zohn, S. R. 1959 Multiple Fourier analysis in rectifier problems. Quart. Appl. Math. 16, 335–360.Google Scholar
[9]Sternberg, R. L., Sheets, M., Sternberg, H. M., Shigematsu, A. & Sternberg, A. L. 1974 Multiple Fourier analysis in rectifier problems II.. Quart. Appl. Math. 31, 293–315.Google Scholar
[10]sternberg, R. L. & Sternberg, H. M. 1976 Backward recurrence relations for Bennett functions. Portugaliae Math. 35, 61–64Google Scholar
[11]Lampard, D. G. 1952 Harmonic intermodulation distortion in power law devices. Proc. IEE IV. 55Google Scholar
[12]Feuerstein, E. 1957 Intermodulation products for v-law biased wave rectifier for multiple frequency input. Quart. Appl. Math. 15, 183–192.Google Scholar
[14]Montroll, E. W. 1954 Frequency spectrum of vibrations of crystal lattices. Am. Math. Month 61 (11), 46–73.Google Scholar
[15]Shipman, J. S. 1955 On Middleton's Paper-some general results in the theory of noise through non-linear devices. Quart. Appl. Math. 13, 200–201.Google Scholar
[16]Hood, M. J.. On the computation of modified Bennett functions. Euro. J. Appl. Math. 3, 93–95.Google Scholar

