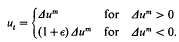Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Hulshof, Josephus
and
Vazquez, Juan Luis
1996.
Maximal viscosity solutions of the modified porous medium equation and their asymptotic behaviour.
European Journal of Applied Mathematics,
Vol. 7,
Issue. 5,
p.
453.
Hulshof, Josephus
1997.
Self-Similar Solutions of Barenblatt's Model for Turbulence.
SIAM Journal on Mathematical Analysis,
Vol. 28,
Issue. 1,
p.
33.
van Dijke, Marinus I. J.
and
van der Zee, Sjoerd E. A. T. M.
1997.
Progress in Industrial Mathematics at ECMI 96.
p.
27.
de Pablo, Arturo
and
Sánchez, Ariel
2000.
Global Travelling Waves in Reaction–Convection–Diffusion Equations.
Journal of Differential Equations,
Vol. 165,
Issue. 2,
p.
377.
Budd, C.J.
and
Piggott, M.D.
2001.
Partial Differential Equations.
p.
399.
Hulshof, J.
King, J. R.
and
Bowen, M.
2001.
Anomalous Exponents and Dipole Solutions for the Thin Film Equation.
SIAM Journal on Applied Mathematics,
Vol. 62,
Issue. 1,
p.
149.
Budd, C.J.
and
Piggott, M.D.
2001.
The geometric integration of scale-invariant ordinary and partial differential equations.
Journal of Computational and Applied Mathematics,
Vol. 128,
Issue. 1-2,
p.
399.
Hulshof, Josephus
2001.
European Congress of Mathematics.
p.
291.
Wagner, Barbara
2005.
An Asymptotic Approach to Second-kind Similarity Solutions of the Modified Porous-medium Equation.
Journal of Engineering Mathematics,
Vol. 53,
Issue. 3-4,
p.
201.
Huang, Yanghong
and
Bertozzi, Andrea L.
2010.
Self-Similar Blowup Solutions to an Aggregation Equation in $R^n$.
SIAM Journal on Applied Mathematics,
Vol. 70,
Issue. 7,
p.
2582.
Seam, Ngonn
and
Vallet, Guy
2011.
Existence results for nonlinear pseudoparabolic problems.
Nonlinear Analysis: Real World Applications,
Vol. 12,
Issue. 5,
p.
2625.
Yao, Yao
and
Bertozzi, Andrea L.
2013.
Blow-up dynamics for the aggregation equation with degenerate diffusion.
Physica D: Nonlinear Phenomena,
Vol. 260,
Issue. ,
p.
77.
Bauzet, Caroline
Lebon, Frédéric
and
Maitlo, Asghar
2019.
The Neumann problem for a Barenblatt equation with a multiplicative stochastic force and a nonlinear source term.
Nonlinear Differential Equations and Applications NoDEA,
Vol. 26,
Issue. 3,
Bauzet, Caroline
Lebon, Frédéric
Maitlo, Asghar Ali
and
Zimmermann, Aleksandra
2021.
Well-posedness for the coupling of a random heat equation with a multiplicative stochastic Barenblatt equation.
Stochastic Analysis and Applications,
Vol. 39,
Issue. 6,
p.
1095.