Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Oberdieck, Georg
2017.
Gromov–Witten invariants of the Hilbert
schemes of points of a K3 surface.
Geometry & Topology,
Vol. 22,
Issue. 1,
p.
323.
Kiem, Young-Hoon
and
Li, Jun
2018.
Localizing Virtual Structure Sheaves by Cosections.
International Mathematics Research Notices,
Creutzig, Thomas
Duncan, John F R
and
Riedler, Wolfgang
2018.
Self-dual vertex operator superalgebras and superconformal field theory.
Journal of Physics A: Mathematical and Theoretical,
Vol. 51,
Issue. 3,
p.
034001.
Oberdieck, Georg
and
Pixton, Aaron
2018.
Holomorphic anomaly equations and the Igusa cusp form conjecture.
Inventiones mathematicae,
Vol. 213,
Issue. 2,
p.
507.
Oberdieck, Georg
2018.
On reduced stable pair invariants.
Mathematische Zeitschrift,
Vol. 289,
Issue. 1-2,
p.
323.
BRYAN, JIM
and
KOOL, MARTIJN
2019.
DONALDSON–THOMAS INVARIANTS OF LOCAL ELLIPTIC SURFACES VIA THE TOPOLOGICAL VERTEX.
Forum of Mathematics, Sigma,
Vol. 7,
Issue. ,
Choi, Jinwon
van Garrel, Michel
Katz, Sheldon
and
Takahashi, Nobuyoshi
2020.
Log BPS numbers of log Calabi-Yau surfaces.
Transactions of the American Mathematical Society,
Vol. 374,
Issue. 1,
p.
687.
Cota, Cesar Fierro
Klemm, Albrecht
and
Schimannek, Thorsten
2021.
State counting on fibered CY 3-folds and the non-Abelian weak gravity conjecture.
Journal of High Energy Physics,
Vol. 2021,
Issue. 5,
Bae, Younghan
and
Buelles, Tim-Henrik
2021.
Curves on K3 surfaces in divisibility 2.
Forum of Mathematics, Sigma,
Vol. 9,
Issue. ,
Kiem, Young-Hoon
and
Park, Hyeonjun
2021.
Virtual intersection theories.
Advances in Mathematics,
Vol. 388,
Issue. ,
p.
107858.
Oprea, Dragos
and
Pandharipande, Rahul
2022.
Quot schemes of curves and surfaces: virtual classes, integrals, Euler characteristics.
Geometry & Topology,
Vol. 25,
Issue. 7,
p.
3425.
Pietromonaco, Stephen
2022.
G-invariant Hilbert schemes on Abelian surfaces and enumerative geometry of the orbifold Kummer surface.
Research in the Mathematical Sciences,
Vol. 9,
Issue. 1,
Shen, Junliang
and
Yin, Qizheng
2022.
Topology of Lagrangian fibrations and Hodge theory of hyper-Kähler manifolds.
Duke Mathematical Journal,
Vol. 171,
Issue. 1,
Oberdieck, Georg
2022.
Gromov–Witten theory and Noether–Lefschetz theory for holomorphic-symplectic varieties.
Forum of Mathematics, Sigma,
Vol. 10,
Issue. ,
Molcho, S.
Pandharipande, R.
and
Schmitt, J.
2023.
The Hodge bundle, the universal 0-section, and the log Chow ring of the moduli space of curves.
Compositio Mathematica,
Vol. 159,
Issue. 2,
p.
306.
Cota, Cesar Fierro
Mininno, Alessandro
Weigand, Timo
and
Wiesner, Max
2023.
The asymptotic weak gravity conjecture in M-theory.
Journal of High Energy Physics,
Vol. 2023,
Issue. 8,
Oberdieck, Georg
2024.
Multiple cover formulas for K3 geometries, wall-crossing, and Quot schemes.
Geometry & Topology,
Vol. 28,
Issue. 7,
p.
3221.
Nesterov, Denis
2024.
On quasimap invariants of moduli spaces of Higgs bundles.
Moduli,
Vol. 1,
Issue. ,
 $K3$ SURFACES
$K3$ SURFACES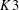 $K3$ surfaces in terms of modular forms. Our results apply in every genus and for every curve class. The proof uses the Gromov–Witten/Pairs correspondence for
$K3$ surfaces in terms of modular forms. Our results apply in every genus and for every curve class. The proof uses the Gromov–Witten/Pairs correspondence for  $K3$ -fibered hypersurfaces of dimension 3 to reduce the KKV conjecture to statements about stable pairs on (thickenings of)
$K3$ -fibered hypersurfaces of dimension 3 to reduce the KKV conjecture to statements about stable pairs on (thickenings of)  $K3$ surfaces. Using degeneration arguments and new multiple cover results for stable pairs, we reduce the KKV conjecture further to the known primitive cases. Our results yield a new proof of the full Yau–Zaslow formula, establish new Gromov–Witten multiple cover formulas, and express the fiberwise Gromov–Witten partition functions of
$K3$ surfaces. Using degeneration arguments and new multiple cover results for stable pairs, we reduce the KKV conjecture further to the known primitive cases. Our results yield a new proof of the full Yau–Zaslow formula, establish new Gromov–Witten multiple cover formulas, and express the fiberwise Gromov–Witten partition functions of  $K3$ -fibered 3-folds in terms of explicit modular forms.
$K3$ -fibered 3-folds in terms of explicit modular forms.