Hostname: page-component-78c5997874-8bhkd Total loading time: 0 Render date: 2024-11-10T21:18:09.467Z Has data issue: false hasContentIssue false
  • English
  • Français

MOMENTS OF RANDOM MULTIPLICATIVE FUNCTIONS, I: LOW MOMENTS, BETTER THAN SQUAREROOT CANCELLATION, AND CRITICAL MULTIPLICATIVE CHAOS

Part of: Probabilistic theory: distribution modulo $1$; metric theory of algorithms Exponential sums and character sums Stochastic processes Multiplicative number theory

Published online by Cambridge University Press:  20 January 2020

ADAM J. HARPER
ADAM J. HARPER*
Affiliation:
Mathematics Institute, Zeeman Building, University of Warwick, CoventryCV4 7AL, England, UK; A.Harper@warwick.ac.uk

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We determine the order of magnitude of 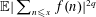$\mathbb{E}|\sum _{n\leqslant x}f(n)|^{2q}$, where $f(n)$ is a Steinhaus or Rademacher random multiplicative function, and 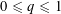$0\leqslant q\leqslant 1$. In the Steinhaus case, this is equivalent to determining the order of 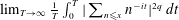$\lim _{T\rightarrow \infty }\frac{1}{T}\int _{0}^{T}|\sum _{n\leqslant x}n^{-it}|^{2q}\,dt$.

In particular, we find that 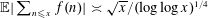$\mathbb{E}|\sum _{n\leqslant x}f(n)|\asymp \sqrt{x}/(\log \log x)^{1/4}$. This proves a conjecture of Helson that one should have better than squareroot cancellation in the first moment and disproves counter-conjectures of various other authors. We deduce some consequences for the distribution and large deviations of 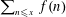$\sum _{n\leqslant x}f(n)$.

The proofs develop a connection between 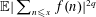$\mathbb{E}|\sum _{n\leqslant x}f(n)|^{2q}$ and the 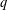$q$th moment of a critical, approximately Gaussian, multiplicative chaos and then establish the required estimates for that. We include some general introductory discussion about critical multiplicative chaos to help readers unfamiliar with that area.

MSC classification

Primary: 11N37: Asymptotic results on arithmetic functions
Secondary: 11L40: Estimates on character sums 11K65: Arithmetic functions 60G15: Gaussian processes 60G57: Random measures
Type
Number Theory
Information
Forum of Mathematics, Pi , Volume 8 , 2020 , e1
Check for updates
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author 2020

References

Arguin, L.-P., Belius, D. and Harper, A. J., ‘Maxima of a randomized Riemann zeta function, and branching random walks’, Ann. Appl. Probab. 27(1) (2017), 178–215.CrossRefGoogle Scholar
Barral, J., Kupiainen, A., Nikula, M., Saksman, E. and Webb, C., ‘Basic properties of critical lognormal multiplicative chaos’, Ann. Probab. 43(5) (2015), 2205–2249.CrossRefGoogle Scholar
Berestycki, N., ‘An elementary approach to Gaussian multiplicative chaos’, Electron. Commun. Probab. 22(Paper No. 27) (2017), 12 pp.CrossRefGoogle Scholar
Bondarenko, A. and Seip, K., ‘Helson’s problem for sums of a random multiplicative function’, Mathematika 62(1) (2016), 101–110.CrossRefGoogle Scholar
Chatterjee, S. and Soundararajan, K., ‘Random multiplicative functions in short intervals’, Int. Math. Res. Not. IMRN 2012 (2012), 479–492.CrossRefGoogle Scholar
Duplantier, B., Rhodes, R., Sheffield, S. and Vargas, V., ‘Renormalization of critical Gaussian multiplicative chaos and KPZ relation’, Comm. Math. Phys. 330(1) (2014), 283–330.CrossRefGoogle Scholar
Grimmett, G. R. and Stirzaker, D. R., Probability and Random Processes, 3rd edn, (Oxford University Press, New York, 2001).Google Scholar
Gut, A., Probability: A Graduate Course, 2nd edn, (Springer Texts in Statistics, New York, 2013).CrossRefGoogle Scholar
Halász, G., ‘On random multiplicative functions’, inHubert Delange Colloquium, (Orsay, 1982), Publications Mathématiques d’Orsay, 83 (Univ. Paris XI, Orsay, 1983), 74–96.Google Scholar
Harper, A. J., ‘Bounds on the suprema of Gaussian processes, and omega results for the sum of a random multiplicative function’, Ann. Appl. Probab. 23(2) (2013), 584–616.CrossRefGoogle Scholar
Harper, A. J., ‘On the limit distributions of some sums of a random multiplicative function’, J. reine angew. Math. 678 (2013), 95–124.Google Scholar
Harper, A. J., ‘Moments of random multiplicative functions, II: High moments’, Algebra Number Theory, to appear.Google Scholar
Harper, A. J., Nikeghbali, A. and Radziwiłł, M., ‘A note on Helson’s conjecture on moments of random multiplicative functions’, inAnalytic Number Theory (Springer, Cham, 2015), 145–169.CrossRefGoogle Scholar
Heap, W. and Lindqvist, S., ‘Moments of random multiplicative functions and truncated characteristic polynomials’, Q. J. Math. 67(4) (2016), 683–714.Google Scholar
Helson, H., ‘Hankel forms’, Studia Math. 198(1) (2010), 79–84.CrossRefGoogle Scholar
Hough, B., ‘Summation of a random multiplicative function on numbers having few prime factors’, Math. Proc. Cambridge Philos. Soc. 150 (2011), 193–214.CrossRefGoogle Scholar
Hu, Y. and Shi, Z., ‘Minimal position and critical martingale convergence in branching random walks, and directed polymers on disordered trees’, Ann. Probab. 37(2) (2009), 742–789.CrossRefGoogle Scholar
Lau, Y.-K., Tenenbaum, G. and Wu, J., ‘On mean values of random multiplicative functions’, Proc. Amer. Math. Soc. 141 (2013), 409–420. Also see www.iecl.univ-lorraine.fr/∼Gerald.Tenenbaum/PUBLIC/Prepublications_et_publications/RMF.pdf for some corrections to the published version.CrossRefGoogle Scholar
Lawler, G. F. and Limic, V., Random Walk: a Modern Introduction, 1st edn, (Cambridge University Press, Cambridge, 2010).CrossRefGoogle Scholar
Montgomery, H. L. and Vaughan, R. C., Multiplicative Number Theory I: Classical Theory, 1st edn, (Cambridge University Press, Cambridge, 2007).Google Scholar
Ng, N., ‘The distribution of the summatory function of the Möbius function’, Proc. Lond. Math. Soc. (3) 89(3) (2004), 361–389.CrossRefGoogle Scholar
Reinert, G. and Röllin, A., ‘Multivariate normal approximation with Stein’s method of exchangeable pairs under a general linearity condition’, Ann. Probab. 37(6) (2009), 2150–2173.CrossRefGoogle Scholar
Rhodes, R. and Vargas, V., ‘Gaussian multiplicative chaos and applications: A review’, Probab. Surv. 11 (2014), 315–392.CrossRefGoogle Scholar
Sadikova, S. M., ‘Two-dimensional analogues of an inequality of Esseen with applications to the Central Limit Theorem’, Theory Probab. Appl. 11 (1966), 325–335.CrossRefGoogle Scholar
Saksman, E. and Seip, K., ‘Integral means and boundary limits of Dirichlet series’, Bull. Lond. Math. Soc. 41(3) (2009), 411–422.CrossRefGoogle Scholar
Saksman, E. and Seip, K., ‘Some open questions in analysis for Dirichlet series’, inRecent Progress on Operator Theory and Approximation in Spaces of Analytic Functions, Contemp. Mathematics, 679 (American Mathematical Society, Providence, RI, 2016), 179–191.CrossRefGoogle Scholar
Saksman, E. and Webb, C., ‘Multiplicative chaos measures for a random model of the Riemann zeta function’, Preprint available online at http://arxiv.org/abs/1604.08378.Google Scholar
Saksman, E. and Webb, C., ‘The Riemann zeta function and Gaussian multiplicative chaos: statistics on the critical line’, Preprint available online at https://arxiv.org/abs/1609.00027.Google Scholar
Soundararajan, K., ‘Moments of the Riemann zeta function’, Ann. of Math. 170 (2009), 981–993.CrossRefGoogle Scholar
Weber, M. J. G., ‘L 1 -Norm of Steinhaus chaos on the polydisc’, Monatsh. Math. 181(2) (2016), 473–483.CrossRefGoogle Scholar
Wintner, A., ‘Random factorizations and Riemann’s hypothesis’, Duke Math. J. 11 (1944), 267–275.CrossRefGoogle Scholar