Published online by Cambridge University Press: 25 March 2020
We prove in generic situations that the lattice in a tame type induced by the completed cohomology of a  $U(3)$-arithmetic manifold is purely local, that is, only depends on the Galois representation at places above
$U(3)$-arithmetic manifold is purely local, that is, only depends on the Galois representation at places above 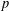 $p$. This is a generalization to
$p$. This is a generalization to 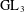 $\text{GL}_{3}$ of the lattice conjecture of Breuil. In the process, we also prove the geometric Breuil–Mézard conjecture for (tamely) potentially crystalline deformation rings with Hodge–Tate weights
$\text{GL}_{3}$ of the lattice conjecture of Breuil. In the process, we also prove the geometric Breuil–Mézard conjecture for (tamely) potentially crystalline deformation rings with Hodge–Tate weights  $(2,1,0)$ as well as the Serre weight conjectures of Herzig [‘The weight in a Serre-type conjecture for tame
$(2,1,0)$ as well as the Serre weight conjectures of Herzig [‘The weight in a Serre-type conjecture for tame  $n$-dimensional Galois representations’, Duke Math. J. 149(1) (2009), 37–116] over an unramified field extending the results of Le et al. [‘Potentially crystalline deformation 3985 rings and Serre weight conjectures: shapes and shadows’, Invent. Math. 212(1) (2018), 1–107]. We also prove results in modular representation theory about lattices in Deligne–Lusztig representations for the group
$n$-dimensional Galois representations’, Duke Math. J. 149(1) (2009), 37–116] over an unramified field extending the results of Le et al. [‘Potentially crystalline deformation 3985 rings and Serre weight conjectures: shapes and shadows’, Invent. Math. 212(1) (2018), 1–107]. We also prove results in modular representation theory about lattices in Deligne–Lusztig representations for the group 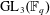 $\text{GL}_{3}(\mathbb{F}_{q})$.
$\text{GL}_{3}(\mathbb{F}_{q})$.