Hostname: page-component-78c5997874-8bhkd Total loading time: 0 Render date: 2024-11-10T13:00:05.473Z Has data issue: false hasContentIssue false
  • English
  • Français

The classification of symmetry protected topological phases of one-dimensional fermion systems

Part of: Selfadjoint operator algebras Groups and algebras in quantum theory

Published online by Cambridge University Press:  16 March 2021

Chris Bourne  and
Yoshiko Ogata
Chris Bourne
Affiliation:
WPI-AIMR, Tohoku University, 2-1-1 Katahira, Aoba-ku, Sendai, 980-8577, Japan. RIKEN iTHEMS, Wako, Saitama351-0198, Japan; E-mail: chris.bourne@tohoku.ac.jp.
Yoshiko Ogata
Affiliation:
Graduate School of Mathematical Sciences, The University of Tokyo, Komaba, Tokyo, 153-8914, Japan; E-mail: yoshiko@ms.u-tokyo.ac.jp.

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

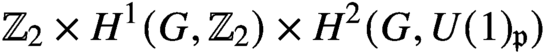We introduce an index for symmetry-protected topological (SPT) phases of infinite fermionic chains with an on-site symmetry given by a finite group G. This index takes values in $\mathbb {Z}_2 \times H^1(G,\mathbb {Z}_2) \times H^2(G, U(1)_{\mathfrak {p}})$ with a generalised Wall group law under stacking. We show that this index is an invariant of the classification of SPT phases. When the ground state is translation invariant and has reduced density matrices with uniformly bounded rank on finite intervals, we derive a fermionic matrix product representative of this state with on-site symmetry.

MSC classification

Primary: 81R15: Operator algebra methods
Secondary: 46L30: States
Type
Mathematical Physics
Information
Forum of Mathematics, Sigma , Volume 9 , 2021 , e25
Check for updates
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence(http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author(s), 2021. Published by Cambridge University Press

References

Affleck, I., Kennedy, T., Lieb, E. H. and Tasaki, H., ‘Valence bond ground states in isotropic quantum antiferromagnets’, Commun. Math. Phys. 115 (1988), 477–528.CrossRefGoogle Scholar
Araki, H. and Moriya, H., ‘Equilibrium statistical mechanics of fermion lattice systems’, Rev. Math. Phys. 15(2) (2003), 93–198.CrossRefGoogle Scholar
Bachmann, S., Michalakis, S., Nachtergaele, B. and Sims, R., ‘Automorphic equivalence of gapped phases of quantum lattice systems’, Commun. Math. Phys. 309(3) (2012), 835–871.CrossRefGoogle Scholar
Bourne, C. and Schulz-Baldes, H., ‘On ${\mathbb{Z}}_2$-indices for ground states of fermionic chains’, Rev. Math. Phys. 32 (2020), 2050028.CrossRefGoogle Scholar
Bratelli, O. and Robinson, D. R., Operators Algebras and Quantum Statistical Mechanics 1, 2nd ed. (Springer, Berlin, 1997).CrossRefGoogle Scholar
Bratelli, O. and Robinson, D. R., Operators Algebras and Quantum Statistical Mechanics 2, 2nd ed. (Springer, Berlin, 1997).CrossRefGoogle Scholar
Bratteli, O., Jorgensen, P. and Price, G., Endomorphisms of $\mathcal{B}(\mathcal{H})$ Quantization, nonlinear partial differential equations, and operator algebra (Cambridge, MA, 1994), 93–138, Proc. Sympos. Pure Math., 59, Amer. Math. Soc., Providence, RI, 1996.Google Scholar
Bratteli, O. and Jorgensen, P. E. T., ‘Endomorphisms of $\mathrm{B}\left(\mathrm{H}\right)$II. Finitely correlated states on ${\mathrm{O}}_{\mathrm{n}}$’, J. Funct. Anal. 145 (1997), 323–373.CrossRefGoogle Scholar
Bratteli, O., Jorgensen, P. E. T., Kishimoto, A. and Werner, R. F., ‘Pure states on ${\mathbf{\mathcal{O}}}_{\mathrm{d}}$’, J. Operator Theory 43 (2000), 97–143.Google Scholar
Bru, J.-B. and de Siqueira Pedra, W., Lieb-Robinson Bounds for Multi-Commutators and Applications to Response Theory . Vol. 13 of Springer Briefs in Mathematical Physics (Springer, Berlin, 2017).CrossRefGoogle Scholar
Bultinck, N., Williamson, D. J., Haegeman, J. and Verstraete, F., ‘Fermionic matrix product states and one-dimensional topological phases’, Phys. Rev. B 95 (2017), 075108.CrossRefGoogle Scholar
Chen, X., Gu, Z.-C. and Wen, X.-G., ‘Classification of gapped symmetric phases in one-dimensional spin systems’, Phys. Rev. B 83 (2011), 035107.Google Scholar
Fannes, M., Nachtergaele, B. and Werner, R. F., ‘Finitely correlated states on quantum spin chains’, Commun. Math. Phys. 144 (1992), 443–490.CrossRefGoogle Scholar
Fidkowski, L. and Kitaev, A., ‘The effects of interactions on the topological classification of free fermion systems’, Phys. Rev. B 81 (2009), 134509.Google Scholar
Fidkowski, L. and Kitaev, A., ‘Topological phases of fermions in one dimension’, Phys. Rev. B 83 (2011), 075103.CrossRefGoogle Scholar
Gu, Z.-C. and Wen, X.-G., ‘Tensor-entanglement-filtering renormalization approach and symmetry-protected topological order’, Phys. Rev. B 80 (2009), 155131.CrossRefGoogle Scholar
Hastings, M., ‘An area law for one-dimensional quantum systems’, J. Stat. Mech. Theory Exp. 2007, no. 8, P08024, 14 pp.CrossRefGoogle Scholar
Kadison, R. V. and Ringrose, J. R., Fundamentals of the Theory of Operator Algebras II. Vol. 16 of Graduate Studies in Mathematics (American Mathematical Society, Providence, RI, 1997).Google Scholar
Kapustin, A. and Thorngren, R., ‘Fermionic SPT phases in higher dimensions and bosonization’, J. High Energy Phys. 2017, no. 10, 080, front matter+48 pp.CrossRefGoogle Scholar
Kapustin, A., Turzillo, A. and You, M., ‘Spin topological field theory and fermionic matrix product states’, Phys. Rev. B 98 (2018), 125101.CrossRefGoogle Scholar
Matsui, T., ‘A characterization of pure finitely correlated states’, Infin. Dimens. Anal. Quantum Probab. Relat. Top. 1(4) (1998), 647–661.CrossRefGoogle Scholar
Matsui, T., ‘The split property and the symmetry breaking of the quantum spin chain’, Commun. Math. Phys. 218 (2001), 393–416.CrossRefGoogle Scholar
Matsui, T., ‘Boundedness of entanglement entropy and split property of quantum spin chains’, Rev. Math. Phys. 26(9) (2013), 1350017.Google Scholar
Matsui, T., ‘Split property and fermionic string order’, 2020, arXiv:2003.13778.Google Scholar
Moon, A. and Ogata, Y., ‘Automorphic equivalence within gapped phases in the bulk’, J. Funct. Anal. 278(8) (2020), 108422.CrossRefGoogle Scholar
Moutuou, M. E., ‘Graded Brauer groups of a groupoid with involution’, J. Funct. Anal. 266(5) (2014), 2689–2739.CrossRefGoogle Scholar
Nachtergaele, B., Sims, R. and Young, A., ‘Lieb-Robinson bounds, the spectral flow, and stability of the spectral gap for lattice fermion systems’, in Mathematical Problems in Quantum Physics, Vol. 717 of Contemp. Math. (American Mathematical Society, Providence, RI, 2018).Google Scholar
Nachtergaele, B., Sims, R. and Young, A., ‘Quasi-locality bounds for quantum lattice systems. Part 1. Lieb-Robinson bounds, quasi-local maps, and spectral flow automorphisms’, J. Math. Phys. 60 (2019), 061101.CrossRefGoogle Scholar
Ogata, Y., ‘A class of asymmetric gapped Hamiltonians on quantum spin chains and its characterization II’, Commun. Math. Phys. 348 (2016), 897–957.CrossRefGoogle Scholar
Ogata, Y., ‘A ${\mathbb{Z}}_2$-index of symmetry protected topological phases with reflection symmetry for quantum spin chains’, 2019, arXiv:1904.01669.CrossRefGoogle Scholar
Ogata, Y., ‘A classification of pure states on quantum spin chains satisfying the split property with on-site finite group symmetries’, 2019, arXiv:1908.08621.Google Scholar
Ogata, Y., ‘A ${\mathbb{Z}}_2$-index of symmetry protected topological phases with time reversal symmetry for quantum spin chains’, Commun. Math. Phys. 374(2) (2020), 705–734.CrossRefGoogle Scholar
Ogata, Y., Tachikawa, Y. and Tasaki, H., ‘General Lieb-Schultz-Mattis type theorems for quantum spin chains’, 2020, arXiv:2004.06458.CrossRefGoogle Scholar
Ogata, Y. and Tasaki, H., ‘Lieb-Schultz-Mattis type theorems for quantum spin chains without continuous symmetry’, Commun. Math. Phys. 372(3)(2019), 951–962.CrossRefGoogle Scholar
Perez-Garcia, D., Wolf, M. M., Sanz, M., Verstraete, F. and Cirac, J. I., ‘String order and symmetries in quantum spin lattices’, Phys. Rev. Lett. 100 (2008), 167202.CrossRefGoogle ScholarPubMed
Pollmann, F., Turner, A., Berg, E. and Oshikawa, M., ‘Entanglement spectrum of a topological phase in one dimension’, Phys. Rev. B 81 (2010), 064439.CrossRefGoogle Scholar
Perez-Garcia, D., Wolf, M. M., Sanz, M., Verstraete, F. and Cirac, J. I., ‘String order and symmetries in quantum spin lattices’, Phys. Rev. Lett. 100 (2008), 167202.CrossRefGoogle ScholarPubMed
Takesaki, M., Theory of Operator Algebras. I. Encyclopaedia of Mathematical Sciences (Springer-Verlag, Berlin, 2002).Google Scholar
Turzillo, A. and You, M., ‘Fermionic matrix product states and one-dimensional short-range entangled phases with antiunitary symmetries’, Phys. Rev. B 99 (2019), 035103.CrossRefGoogle Scholar
Wall, C. T. C., ‘Graded Brauer groups’, J. Reine Angew. Math. 213 (1963–1964), 187–199.Google Scholar