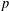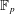Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Nizioł, Wiesława
2020.
On uniqueness ofp-adic period morphisms, II.
Compositio Mathematica,
Vol. 156,
Issue. 9,
p.
1915.
Diao, Hansheng
Lan, Kai-Wen
Liu, Ruochuan
and
Zhu, Xinwen
2022.
Logarithmic Riemann–Hilbert correspondences for rigid varieties.
Journal of the American Mathematical Society,
Vol. 36,
Issue. 2,
p.
483.