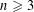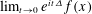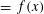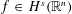Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Cao, Zhenbin
Fan, Dashan
and
Wang, Meng
2018.
The rate of convergence on Schrödinger operator.
Illinois Journal of Mathematics,
Vol. 62,
Issue. 1-4,
Li, Dan
and
Li, Junfeng
2019.
On 4-order Schrödinger operator and Beam operator.
Frontiers of Mathematics in China,
Vol. 14,
Issue. 6,
p.
1197.
Pierce, Lillian B
2020.
On Bourgain’s Counterexample for the Schrödinger Maximal Function.
The Quarterly Journal of Mathematics,
Vol. 71,
Issue. 4,
p.
1309.
Compaan, Erin
Lucà, Renato
and
Staffilani, Gigliola
2020.
Pointwise Convergence of the Schrödinger Flow.
International Mathematics Research Notices,
Vol. 2021,
Issue. 1,
p.
596.
Li, Dan
and
Yu, Haixia
2020.
Convergence of a class of Schrödinger equations.
Rocky Mountain Journal of Mathematics,
Vol. 50,
Issue. 2,
Lee, Jungjin
2020.
Global Kato Type Smoothing Estimates via Local Ones for Dispersive Equations.
Journal of Fourier Analysis and Applications,
Vol. 26,
Issue. 1,
Wang, Junfang
Yan, Wei
and
Yan, Xiangqian
2021.
Probabilistic pointwise convergence problem of Schrödinger equations on manifolds.
Proceedings of the American Mathematical Society,
Vol. 149,
Issue. 8,
p.
3367.
Li, Dan
Li, Junfeng
and
Xiao, Jie
2021.
An Upbound of Hausdorff’s Dimension of the Divergence Set of the Fractional SchröDinger Operator On Hs(ℝn).
Acta Mathematica Scientia,
Vol. 41,
Issue. 4,
p.
1223.
Cao, Zhenbin
Miao, Changxing
and
Wang, Meng
2021.
L-estimate of Schrödinger maximal function in higher dimensions.
Journal of Functional Analysis,
Vol. 281,
Issue. 5,
p.
109091.
Wu, Shukun
2021.
A Note on the Refined Strichartz Estimates and Maximal Extension Operator.
Journal of Fourier Analysis and Applications,
Vol. 27,
Issue. 3,
Yan, Wei
Wang, Weimin
and
Yan, Xiangqian
2022.
Convergence problem of the Kawahara equation on the real line.
Journal of Mathematical Analysis and Applications,
Vol. 515,
Issue. 1,
p.
126386.
Eceizabarrena, Daniel
and
Lucà, Renato
2022.
Convergence over fractals for the periodic
Schrödinger equation.
Analysis & PDE,
Vol. 15,
Issue. 7,
p.
1775.
Yan, Xiangqian
Zhao, Yajuan
and
Yan, Wei
2022.
Convergence problem of Schrödinger equation in Fourier-Lebesgue spaces with rough data and random data.
Proceedings of the American Mathematical Society,
Cho, Chu-Hee
and
Ko, Hyerim
2022.
Pointwise Convergence of the Fractional Schrödinger Equation in $\mathbb{R}^2$.
Taiwanese Journal of Mathematics,
Vol. 26,
Issue. 1,
Eceizabarrena, Daniel
and
Ponce-Vanegas, Felipe
2022.
Pointwise convergence over fractals for dispersive equations with homogeneous symbol.
Journal of Mathematical Analysis and Applications,
Vol. 515,
Issue. 1,
p.
126385.
Lucà, Renato
2022.
Qualitative Properties of Dispersive PDEs.
Vol. 52,
Issue. ,
p.
61.
Chu, Rena
and
Pierce, Lillian B.
2023.
Generalizations of the Schrödinger maximal operator: building arithmetic counterexamples.
Journal d'Analyse Mathématique,
Vol. 151,
Issue. 1,
p.
59.
Li, Dan
and
Li, Xiang
2023.
A note on Boussinesq maximal estimate.
AIMS Mathematics,
Vol. 9,
Issue. 1,
p.
1819.
Pan, Yali
2023.
Some Convergence Problems Regarding the Fractional Schrödinger Propagator on Noncompact Manifolds.
Acta Mathematica Scientia,
Vol. 43,
Issue. 5,
p.
2309.
Yan, Xiangqian
Yan, Wei
Zhao, Yajuan
and
Yang, Meihua
2023.
Convergence problem of reduced Ostrovsky equation in Fourier–Lebesgue spaces with rough data and random data.
Stochastics and Dynamics,
Vol. 23,
Issue. 01,