No CrossRef data available.
Article contents
Arens regularity and retractions
Published online by Cambridge University Press: 18 May 2009
Extract
In this paper a characterisation of the regularity of a normed algebra A is given in terms of retractions onto A** from A4*. The second dual A** of a normed algebra A possesses two natural Banach algebra multiplications, say ° and *. Each of ° and * extends the original algebra multiplication on A; see (2). An algebra A is called regular if and only if F * G = F ° G for all F, G ∈ A**. See (1). The existing results in the Arens regularity theory can be found in a recent survey (2). Denoting the nth dual of A by An*, and en the natural embedding of An* in its second dual A(n+2)*, we can naturally represent the second dual A** of A as a Banach space retract of A4* in two different ways:
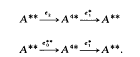
Our main results say that A** is in fact a Banach algebra retract of A4* (i.e. the maps involved are homomorphisms) in either of these cases if and only if A is regular.
- Type
- Research Article
- Information
- Copyright
- Copyright © Glasgow Mathematical Journal Trust 1983


