Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-25T06:59:15.506Z Has data issue: false hasContentIssue false
  • English
  • Français

COMODULES AND CONTRAMODULES

Published online by Cambridge University Press:  24 June 2010

ROBERT WISBAUER
ROBERT WISBAUER*
Affiliation:
Department of Mathematics, Heinrich Heine University, 40225 Düsseldorf, Germany e-mail: wisbauer@math.uni-duesseldorf.de
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Algebras A and coalgebras C over a commutative ring R are defined by properties of the (endo)functors A ⊗R – and C ⊗R – on the category of R-modules R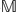. Generalising these notions, monads and comonads were introduced on arbitrary categories, and it turned out that some of their basic relations do not depend on the specific properties of the tensor product. In particular, the adjoint of any comonad is a monad (and vice versa), and hence, for any coalgebra C, HomR(C, –), the right adjoint of C ⊗R –, is a monad on R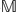. The modules for the monad HomR(C, –) were called contramodules by Eilenberg–Moore and the purpose of this talk is to outline the related constructions and explain the relationship between C-comodules and C-contramodules. The results presented grew out from cooperation with G. Böhm, T. Brzeziński and B. Mesablishvili.

Keywords

16T1518C20
Type
Research Article
Information
Glasgow Mathematical Journal , Volume 52 , Issue A: Rings and Modules in Honour of Patrick F. Smith's 65th Birthday , July 2010 , pp. 151 - 162
Copyright
Copyright © Glasgow Mathematical Journal Trust 2010

References

REFERENCES

1.Böhm, G., Brzeziński, T. and Wisbauer, R., Monads and comonads on module categories, J. Algebra 322 (2009), 1719–1747.CrossRefGoogle Scholar
2.Brzeziński, T. and Wisbauer, R., Corings and comodules, London Mathematical Society Lecture Note Series 309 (Cambridge University Press, Cambridge, UK, 2003).CrossRefGoogle Scholar
3.Dubuc, E., Kan extensions in enriched category theory, Lecture Notes in Mathematics 145 (Springer-Verlag, Berlin, 1970)CrossRefGoogle Scholar
4.Eilenberg, S. and Moore, J. C., Adjoint functors and triples, Ill. J. Math. 9 (1965), 381–398.Google Scholar
5.Mesablishvili, B. and Wisbauer, R., Bimonads and Hopf monads on categories, J. K-theory. doi:10.1017/is010001014jkt105 (2010).Google Scholar
6.Positselski, L., Homological algebra of semimodules and semicontramodules, (Birkhauser, Basel, 2010). arXiv:0708.3398v12.CrossRefGoogle Scholar
7.Wisbauer, R., Hopf monads on categories, in Noncommutative rings, group rings, diagram algebras and their applications, Contemporary Mathematics, vol. 456 (Jain, S. K. et al. , Editors) (American Mathematical Society, Providence, RI, 2008), 219–230.CrossRefGoogle Scholar