Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Thijsse, Philip
1997.
Upper triangular similarity of upper triangular matrices.
Linear Algebra and its Applications,
Vol. 260,
Issue. ,
p.
119.
Vera-López, Antonio
and
Arregi, J.M
2001.
Polynomial Properties in Unitriangular Matrices.
Journal of Algebra,
Vol. 244,
Issue. 1,
p.
343.
Vera-López, Antonio
and
Arregi, J.M.
2003.
Conjugacy classes in unitriangular matrices.
Linear Algebra and its Applications,
Vol. 370,
Issue. ,
p.
85.
Vera-López, Antonio
and
Arregi, J.M
2004.
Computing in unitriangular matrices over finite fields.
Linear Algebra and its Applications,
Vol. 387,
Issue. ,
p.
193.
Vera-López, A.
Arregi, J.M.
Ormaetxea, Leyre
and
Vera-López, F.J.
2008.
The exact number of conjugacy classes of the Sylow p-subgroups of GL(n,q) modulo (q-1)13.
Linear Algebra and its Applications,
Vol. 429,
Issue. 2-3,
p.
617.
Vera-López, A.
García-Sánchez, M.A.
Basova, O.
and
Vera-López, F.J.
2014.
A generalization of Catalan numbers.
Discrete Mathematics,
Vol. 332,
Issue. ,
p.
23.
O’Brien, E.
and
Voll, C.
2015.
Enumerating classes and characters of 𝑝-groups.
Transactions of the American Mathematical Society,
Vol. 367,
Issue. 11,
p.
7775.
Vera-López, A.
and
García-Sánchez, M.A.
2015.
Counting conjugacy classes for unitriangular matrices.
Linear Algebra and its Applications,
Vol. 486,
Issue. ,
p.
275.
Chen, Yuan
Xu, Yunge
Li, Huanhuan
and
Fu, Wenhao
2016.
Belitskii's canonical forms of upper triangular nilpotent matrices under upper triangular similarity.
Linear Algebra and its Applications,
Vol. 506,
Issue. ,
p.
139.
Vera-López, A.
Arregi, J. M.
Ormaetxea, Leyre
Vera-López, F. J.
and
Basova, Olga
2016.
Equalities on the number of conjugacy classes of the Sylow p-subgroups of GL(n, q).
Israel Journal of Mathematics,
Vol. 214,
Issue. 2,
p.
675.
Vera López, Antonio
Martínez, Luis
Vera Pérez, Antonio
Vera Pérez, Beatriz
and
Basova, Olga
2017.
Combinatorics related to Higman's conjecture I: Parallelogramic digraphs and dispositions.
Linear Algebra and its Applications,
Vol. 530,
Issue. ,
p.
414.


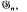 , the Sylow
, the Sylow