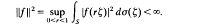Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Ueki, Sei-Ichiro
Luo, Luo
and
Clark, Stephen
2008.
Compact Weighted Composition Operators and Multiplication Operators between Hardy Spaces.
Abstract and Applied Analysis,
Vol. 2008,
Issue. 1,
Lefèvre, Pascal
Li, Daniel
Queffélec, Hervé
and
Rodríguez-Piazza, Luis
2011.
Nevanlinna counting function and Carleson function of analytic maps.
Mathematische Annalen,
Vol. 351,
Issue. 2,
p.
305.
Charpentier, Stéphane
2012.
Essential norm of composition operators on the Hardy space H 1 and the weighted Bergman spaces $${A_{\alpha}^{p}}$$ on the ball.
Archiv der Mathematik,
Vol. 98,
Issue. 4,
p.
327.
Jiang, Liangying
and
Chen, Zhihua
2014.
Spectra of linear fractional composition operators onH2(BN).
Complex Variables and Elliptic Equations,
Vol. 59,
Issue. 9,
p.
1283.
Aleksandrov, Aleksei B.
and
Doubtsov, Evgueni
2020.
Clark measures on the complex sphere.
Journal of Functional Analysis,
Vol. 278,
Issue. 2,
p.
108314.
Clos, Timothy G.
Čučković, Željko
and
Şahutoğlu, Sönmez
2025.
Compactness of Composition Operators on the Bergman Space of the Bidisc.
Integral Equations and Operator Theory,
Vol. 97,
Issue. 3,