Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ono, Ken
1994.
Shimura sums related to imaginary quadratic fields.
Proceedings of the Japan Academy, Series A, Mathematical Sciences,
Vol. 70,
Issue. 5,
Ono, Ken
Robins, Sinai
and
Wahl, Patrick T.
1995.
On the representation of integers as sums of triangular numbers.
Aequationes Mathematicae,
Vol. 50,
Issue. 1-2,
p.
73.
Kahl, Helmut
and
Köhler, Günter
1999.
Components of Hecke Theta Series.
Journal of Mathematical Analysis and Applications,
Vol. 232,
Issue. 2,
p.
312.
Ahlgren, Scott
2001.
Multiplicative Relations in Powers of Euler's Product.
Journal of Number Theory,
Vol. 89,
Issue. 2,
p.
222.
2006.
The Mathematica GuideBook for Symbolics.
p.
978.
Alladi, Krishnaswami
Andrews, George E.
Ono, Ken
and
McIntosh, Richard J.
2006.
On the work of Basil Gordon.
Journal of Combinatorial Theory, Series A,
Vol. 113,
Issue. 1,
p.
21.
Chen, Shi-Chao
2013.
Ramanujan-type congruences for certain generating functions.
Lithuanian Mathematical Journal,
Vol. 53,
Issue. 4,
p.
381.
Voskresenskaya, G. V.
2014.
The Dedekind Eta-Function in Algebra and Number Theory: Old and New Problems.
Journal of Mathematical Sciences,
Vol. 199,
Issue. 3,
p.
248.
Chen, Shi-Chao
2014.
Arithmetic properties of a partition pair function.
International Journal of Number Theory,
Vol. 10,
Issue. 06,
p.
1583.
Williams, Kenneth S.
2015.
Ternary Quadratic Forms and Eta Quotients.
Canadian Mathematical Bulletin,
Vol. 58,
Issue. 4,
p.
858.
Barış Kendirli
2015.
Fourier Coefficients of a Class of Eta Quotients of Weight 14 with Level 12.
Journal of Basic & Applied Sciences,
Vol. 11,
Issue. ,
p.
454.
Kendirli, Barış
2015.
Fourier Coefficients of a Class of Eta Quotients of Weight 16 with Level 12.
Applied Mathematics,
Vol. 06,
Issue. 08,
p.
1426.
Voskresenskaya, G. V.
2018.
Dedekind η-Function in Modern Research.
Journal of Mathematical Sciences,
Vol. 235,
Issue. 6,
p.
788.
Wagner, Ian
2023.
Jacobi forms with CM and applications.
Journal of Number Theory,
Vol. 246,
Issue. ,
p.
15.
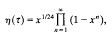

 with a multiplier system. The Fourier coefficients of η-products are related to many well-known number-theoretic functions, including partition functions and quadratic form representation numbers. They also arise from representations of the “monster” group [3] and the Mathieu group M24 [13]. The multiplicative structure of these Fourier coefficients has been extensively studied. Recent papers include [1], [4], [5] and [6]. Here we study the connections between the density of the non-zero Fourier coefficients of f(τ) and the representability of f(τ) as a linear combination of Hecke character forms (defined in Section 4 below). We first make the following definition.
with a multiplier system. The Fourier coefficients of η-products are related to many well-known number-theoretic functions, including partition functions and quadratic form representation numbers. They also arise from representations of the “monster” group [3] and the Mathieu group M24 [13]. The multiplicative structure of these Fourier coefficients has been extensively studied. Recent papers include [1], [4], [5] and [6]. Here we study the connections between the density of the non-zero Fourier coefficients of f(τ) and the representability of f(τ) as a linear combination of Hecke character forms (defined in Section 4 below). We first make the following definition.