Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-15T12:01:29.808Z Has data issue: false hasContentIssue false
  • English
  • Français

Matrix invariants and complete intersections

Published online by Cambridge University Press:  18 May 2009

Lieven le Bruyn  and
Yasuo Teranishi
Lieven le Bruyn
Affiliation:
University of AntwerpUIA-NFWO
Yasuo Teranishi
Affiliation:
University of MannheimFRG and University of Nagoya, Japan
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Consider the vector space of m-tuples of n by n matrices

.

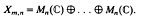The linear group GLn(C) acts on Xm, n by simultaneous conjugation. The corresponding ring of polynomial invariants

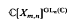
will be denoted by C(n, m) and is called the ring of matrix invariants of m-tuples of n by n matrices. C. Procesi has shown in [8] that C(n, m) is generated by traces of products of the corresponding generic matrices and, as such, coincides with the center of the trace ring of m generic n by n matrices R (n, m) which is also the ring of equivariant maps from Xm, n to Mn(ℂ).

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 32 , Issue 2 , May 1990 , pp. 227 - 229
Copyright
Copyright © Glasgow Mathematical Journal Trust 1990

References

REFERENCES

0.Formanek, E., Invariants and the ring of generic matrices, J. Algebra 89 (1984), 178–223.CrossRefGoogle Scholar
1.le Bruyn, L., The Artin-Schofield theorem and some applications, Comm. Algebra 14 (1986), 1439–1455.Google Scholar
2.le Bruyn, L., Trace rings of generic 2 by 2 matrices, Mem. Amer. Math. Soc. 363 (1987).Google Scholar
3.le Bruyn, L. and Bergh, M. van den, An explicit description of π3,2, Ring theory (Ed. Oystaeyen, F. M. J. van), Lecture Notes in Mathematics No. 1197 (Springer, 1986), 109–113.CrossRefGoogle Scholar
4.le Bruyn, L. and Bergh, M. van den, Regularity of trace rings of generic matrices, J. Algebra 117 (1988), 19–29.Google Scholar
5.le Bruyn, L. and Procesi, C., Etale local structure of matrix invariants and concomitants, Algebraic groups, Utrecht 1986 (Ed. Cohen, A. M., Hesselink, W. H., Kallen, W. L. J. van der and Strooker, J. R.), Lecture Notes in Mathematics 1271 (Springer, 1987), 143–175.Google Scholar
6.le Bruyn, L. and Procesi, C., Semi-simple representations of quivers, Trans. Amer. Math. Soc. to appear.Google Scholar
7.Procesi, C., Rings with polynomial identities (Marcel Dekker, 1973).Google Scholar
8.Procesi, C., Invariant theory of n × n matrices, Adv. in Math. 19 (1976), 306–381.Google Scholar
9.Procesi, C., Computing with 2 × 2 matrices, Algebra 87 (1984), 342–359.Google Scholar
10.Teranishi, Y., The ring of invariants of matrices, Nagoya Math. J. 104 (1986), 149–161.CrossRefGoogle Scholar