Hostname: page-component-7f64f4797f-tldsr Total loading time: 0.001 Render date: 2025-11-04T01:26:46.921Z Has data issue: false hasContentIssue false
  • English
  • Français

Multiplicative forms and nonassociative algebras

Published online by Cambridge University Press:  18 May 2009

R. W. K. Odoni
R. W. K. Odoni
Affiliation:
University Of Glasgow, Glasgow, G12 8QW
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In [1], we introduced the notion of multiplicative forms on associative algebras 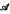of finite rank over integral domains D, and obtained a complete classification when D ⊆, the complex field. We propose here to remove the hypothesis of associativity, using a refinement of the technique of Schafer [2]. In [l], it was noted that multiplicative forms extend uniquely under the adjunction of an identity when 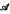is associative but not unitary; this appears difficult to verify in the general case, so that some mild restriction on 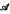is required. We shall assume that 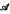is biregular, that is that 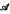contains elements eL, eR such that the linear maps x 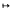eL x and x 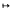xeR, are bijective on 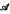We can then (§1) reduce the biregular case to the unitary case, which is handled in §2.

Information

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 15 , Issue 2 , September 1974 , pp. 129 - 130
Copyright
Copyright © Glasgow Mathematical Journal Trust 1974

References

REFERENCES

1.Odoni, R., Multiplicative forms and associative algebras, J. London Math. Soc.; to appear.Google Scholar
2.Schafer, R. D., On forms of degree n permitting composition, J. Math, and Mech. 12 (1963), 777–792.Google Scholar
3.Jacobson, N., The generic norm of an algebra, Osaka Math. J. 15 (1963), 25–50.Google Scholar
4.Schafer, R. D., An introduction to nonassociative algebras (New York, 1966).Google Scholar