No CrossRef data available.
Published online by Cambridge University Press: 02 August 2012
We prove, using purely combinatorial methods, that there is a pairing
with an M2(ℚ)-equivariance property.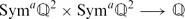 \begin{linenomath}
$$\Sym^a\Q^2\times\Sym^a\Q^2\lra\Q$$
\end{linenomath}
\begin{linenomath}
$$\Sym^a\Q^2\times\Sym^a\Q^2\lra\Q$$
\end{linenomath}