No CrossRef data available.
Article contents
On the approximation of π by special algebraic numbers
Published online by Cambridge University Press: 18 May 2009
Extract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Suppose that m0 is an integer, m0≥3, ρ = exp(2πi/m0), K = ℚ(ρ, i), v denotes the degree of K, ξ∈K has degree N over ℚ. The length, 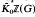 where
where 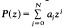 is the (irreducible) minimal polynomial for with ξ relatively prime integer coefficients. Feldman [2, p. 49] proved that there is an absolute constant c0>0 such that
is the (irreducible) minimal polynomial for with ξ relatively prime integer coefficients. Feldman [2, p. 49] proved that there is an absolute constant c0>0 such that
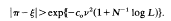
From [2, p. 49, Notes 1 and 2] we know that v = φ(m0) or v = 2φ(m0), and φ(m0)≥ c1m0(log log m0)−1 (c1 > an absolute constant), where φ(m0) denotes Euler's function.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Glasgow Mathematical Journal Trust 1980
References
REFERENCES
1.Cijsouw, P. L., A transcendence measure for π, Transcendence theory: advances and applications, Proc. of a conference in Cambridge 1976 (Ed. Baker, A. and Masser, D. W.), (Academic Press, 1977).Google Scholar
2.Feldman, N. I., Approximation of number π by algebraic numbers from special fields, J. Number Theory 9 (1977), 48–60.CrossRefGoogle Scholar

