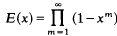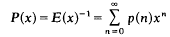Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Alladi, Krishnaswami
Andrews, George E.
Ono, Ken
and
McIntosh, Richard J.
2006.
On the work of Basil Gordon.
Journal of Combinatorial Theory, Series A,
Vol. 113,
Issue. 1,
p.
21.
BARUAH, NAYANDEEP DEKA
and
OJAH, KANAN KUMARI
2011.
SOME CONGRUENCES DEDUCIBLE FROM RAMANUJAN'S CUBIC CONTINUED FRACTION.
International Journal of Number Theory,
Vol. 07,
Issue. 05,
p.
1331.
Paule, Peter
and
Radu, Cristian-Silviu
2012.
The Andrews–Sellers family of partition congruences.
Advances in Mathematics,
Vol. 230,
Issue. 3,
p.
819.
Andrews, George E.
and
Berndt, Bruce C.
2012.
Ramanujan's Lost Notebook.
p.
181.
Narkiewicz, Władysław
2012.
Rational Number Theory in the 20th Century.
p.
13.
Baruah, Nayandeep Deka
and
Sarmah, Bipul Kumar
2013.
Identities and congruences for the general partition and Ramanujan’s tau functions.
Indian Journal of Pure and Applied Mathematics,
Vol. 44,
Issue. 5,
p.
643.
Chen, William Y. C.
Du, Daniel K.
Hou, Qing-Hu
and
Sun, Lisa H.
2014.
Congruences of multipartition functions modulo powers of primes.
The Ramanujan Journal,
Vol. 35,
Issue. 1,
p.
1.
Zhao, Tao Yan
Jin, Lily J.
and
Gu, C.
2016.
Some congruences for 3-component multipartitions.
Open Mathematics,
Vol. 14,
Issue. 1,
p.
783.
Tang, Dazhao
2018.
Congruences modulo powers of 5 for k-colored partitions.
Journal of Number Theory,
Vol. 187,
Issue. ,
p.
198.
Du, Julia Q. D.
Liu, Edward Y. S.
and
Zhao, Jack C. D.
2019.
Congruence properties of pk(n).
International Journal of Number Theory,
Vol. 15,
Issue. 06,
p.
1267.
Petta Mestrige, Shashika
2020.
Congruences modulo powers of 11 for some eta-quotients.
Research in Number Theory,
Vol. 6,
Issue. 1,
Xia, Ernest X. W.
and
Zhu, Haiyang
2020.
Proofs of some conjectures of Chan and Wang on congruences for $$(q;q)_\infty ^{\frac{a}{b}}$$.
The Ramanujan Journal,
Vol. 53,
Issue. 2,
p.
245.
Hemanthkumarm, B.
Ranganatha, D.
and
Bharadwaj, H. S. Sumanth
2020.
On Eight Colour Partitions.
Indian Journal of Pure and Applied Mathematics,
Vol. 51,
Issue. 4,
p.
1843.
Srivatsa Kumar, B. R.
Shruthi
and
Ranganatha, D.
2020.
Some New Congruences Modulo 5 for the General Partition Function.
Russian Mathematics,
Vol. 64,
Issue. 7,
p.
73.
Baruah, Nayandeep Deka
and
Das, Hirakjyoti
2021.
Families of congruences for fractional partition functions modulo powers of primes.
Research in Number Theory,
Vol. 7,
Issue. 4,
Baruah, Nayandeep Deka
and
Das, Hirakjyoti
2022.
Infinite families of congruences modulo powers of 2 for some partition functions involving only odd parts.
International Journal of Number Theory,
Vol. 18,
Issue. 08,
p.
1843.
Petta Mestrige, Shashika
2022.
Congruences for a class of eta-quotients and their applications.
The Ramanujan Journal,
Vol. 58,
Issue. 2,
p.
407.
Atmani, Sofiane
Bayad, Abdelmejid
and
Hernane, Mohand Ouamar
2023.
Congruences for Fourier coefficients of eta‐quotients modulo powers of 5, 7, 11, 13, and 17.
Mathematical Methods in the Applied Sciences,
Vol. 46,
Issue. 5,
p.
5001.
Wang, Liuquan
and
Yang, Yifan
2023.
Moments of ranks and cranks, and quotients of Eisenstein series and the Dedekind eta function.
Journal of Number Theory,
Vol. 242,
Issue. ,
p.
471.
Dicks, Robert
2023.
Congruence relations for r-colored partitions.
Journal of Number Theory,
Vol. 249,
Issue. ,
p.
377.