Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Ito, Akiko
2011.
A note on the divisibility of class numbers of imaginary quadratic fields $\mathbf{Q}(\sqrt{a^{2} - k^{n}})$.
Proceedings of the Japan Academy, Series A, Mathematical Sciences,
Vol. 87,
Issue. 9,
Ito, Akiko
2015.
Notes on the divisibility of the class numbers of imaginary quadratic fields $$\mathbb {Q}(\sqrt{3^{2e} - 4k^n})$$ Q ( 3 2 e - 4 k n ).
Abhandlungen aus dem Mathematischen Seminar der Universität Hamburg,
Vol. 85,
Issue. 1,
p.
1.
Chakraborty, Kalyan
Hoque, Azizul
and
Sharma, Richa
2018.
Advances in Mathematical Inequalities and Applications.
p.
247.
Chakraborty, K.
Hoque, A.
Kishi, Y.
and
Pandey, P.P.
2018.
Divisibility of the class numbers of imaginary quadratic fields.
Journal of Number Theory,
Vol. 185,
Issue. ,
p.
339.
Kalita, Himashree
and
Saikia, Helen K.
2020.
Class Groups of Number Fields and Related Topics.
p.
141.
Kim, Kwang-Seob
2022.
Some Remarks on the Divisibility of the Class Numbers of Imaginary Quadratic Fields.
Mathematics,
Vol. 10,
Issue. 14,
p.
2488.
Kim, Kwang-Seob
and
König, Joachim
2024.
On -unramified extensions over imaginary quadratic fields.
Glasgow Mathematical Journal,
Vol. 66,
Issue. 1,
p.
119.
Agnihotri, Rishabh
Laishram, Shanta
and
Mishra, Mohit
2025.
On the class number of $${\mathbb {Q}}(\sqrt{(2^ra)^{2}- y^p})$$.
The Ramanujan Journal,
Vol. 66,
Issue. 1,
Jin, Seokho
and
Kim, Kwang-Seob
2025.
A new family of imaginary quadratic fields with class number divisible by 5.
The Ramanujan Journal,
Vol. 66,
Issue. 3,


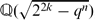 , where
, where 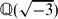 are divisible by
are divisible by 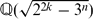 when
when 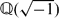 are divisible by
are divisible by 