Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Stothers, W. W.
1981.
Subgroups of infinite index in the modular group III.
Glasgow Mathematical Journal,
Vol. 22,
Issue. 2,
p.
119.
Brenner, J. L.
and
Lyndon, R. C.
1983.
Maximal nonparabolic subgroups of the modular group.
Mathematische Annalen,
Vol. 263,
Issue. 1,
p.
1.
Lyndon, R. C.
1984.
Groups — Korea 1983.
Vol. 1098,
Issue. ,
p.
69.
Kulkarni, Ravi S.
1989.
Geometry of Neumann subgroups.
Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics,
Vol. 47,
Issue. 3,
p.
350.
Hamann, Matthias
2017.
The classification of finite and locally finite connected-homogeneous digraphs.
Combinatorica,
Vol. 37,
Issue. 2,
p.
183.

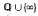 under the action of
under the action of 