Two Notes on Matrices

Extract

1. The properties of the circulant determinant or the circulant matrix are familiar. The circulant matrix C of order 4 x 4, with elements in the complex field, will serve for illustration.

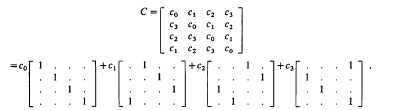
The four matrix coefficients of c₀, c₁ c₂, c₃ form a reducible matrix representation of the cyclic group ℐ₄, so that C is a group matrix for this. Let ω be a primitive 4th root of 1. Then Ω as below, its columns being normalized latent vectors of C,

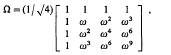
is unitary and symmetric, and reduces Cto diagonal form thus,

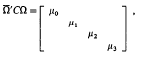
where the μ_r, the latent roots of C, are given by

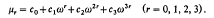
All of the above extends naturally to the n x n case.