Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-15T10:02:06.564Z Has data issue: false hasContentIssue false
  • English
  • Français

Uncountable existentially closed groups in locally finite group classes

Published online by Cambridge University Press:  18 May 2009

Felix Leinen
Felix Leinen
Affiliation:
Fachbereich 17—Mathematik, Johannes Gutenberg Universität, Saarstr. 21, D-6500 Mainz, West Germany.
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this paper, 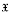will always denote a local class of locally finite groups, which is closed with respect to subgroups, homomorphic images, extensions, and with respect to cartesian powers of finite 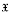-groups. Examples for x are the classes L ℐπ of all locally finite π-groups and L(ℐπ ∩ ) of all locally soluble π-groups (where π is a fixed set of primes). In [4], a wreath product construction was used in the study of existentially closed 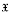-groups (=e.c. 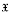-groups); the restrictive type of construction available in [4] permitted results for only countable groups. This drawback was then removed partially in [5] with the help of permutational products. Nevertheless, the techniques essentially only permitted amalgamation of 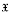-groups with locally nilpotent π-groups. Thus, satisfactory results could be obtained for L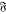p-groups (resp. locally nilpotent π-groups) [6], while the theory remained incomplete in all other cases.

Type
Research Article
Information
Glasgow Mathematical Journal , Volume 32 , Issue 2 , May 1990 , pp. 153 - 163
Copyright
Copyright © Glasgow Mathematical Journal Trust 1990

References

REFERENCES

1.Ensel, H., Existentiell abgeschlossene Sylowturmgruppen, Arch. Math. 51 (1988), 385–392.CrossRefGoogle Scholar
2.Higman, G., Amalgams of p-groups, J. Algebra 1 (1964), 301–305.CrossRefGoogle Scholar
3.Kegel, O. H. and Wehrfritz, B. A. F., Locally finite groups (North-Holland, 1973).Google Scholar
4.Leinen, F., Existentially closed Lx-groups, Rend. Sent. Mat. Univ. Padova 75 (1986), 191–226.Google Scholar
5.Leinen, F., Existentially closed groups in locally finite group classes, Comm. Algebra 13 (1985), 1991–2024.CrossRefGoogle Scholar
6.Leinen, F., Existentially closed locally finite p-groups, Comm. Algebra 103 (1986), 160–183.CrossRefGoogle Scholar
7.Leinen, F., An amalgamation theorem for soluble groups, Canad. Math. Bull. 30 (1987), 9–18.CrossRefGoogle Scholar
8.Leinen, F. and Phillips, R. E., Algebraically closed groups in locally finite group classes, in: Kegel, O. H., Menegazzo, F. and Zacher, G. (ed.) Group theory, Proceedings Brixen/Bressanone 1986, Lecture Notes in Mathematics 1281 (Springer-Verlag 1987), 85–102.Google Scholar
9.Neumann, B. H., On amalgams of periodic groups, Proc. Roy. Soc. London (A) 255 (1960), 477–489.Google Scholar