Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-11T03:40:53.785Z Has data issue: false hasContentIssue false
  • English
  • Français

Incompressible MHD Turbulence

Published online by Cambridge University Press:  12 April 2016

Peter Goldreich
Peter Goldreich*
Affiliation:
California Institute of Technology, 150-21, Pasadena, CA 91125, USA. E-mail: pmg@gps.caltech.edu

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The inertial range of incompressible MHD turbulence is most conveniently described in terms of counter propagating waves. Shear Alfvén waves control the cascade dynamics. Slow waves play a passive role and adopt the spectrum set by the shear Alfvén waves. Cascades composed entirely of shear Alfvén waves do not generate a significant measure of slow waves. MHD turbulence is anisotropic with energy cascading more rapidly along k⊥ than along k‖. Anisotropy increases with k⊥ such that the excited modes are confined inside a cone bounded by 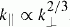. The opening angle of the cone, 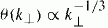, defines the scale dependent anisotropy. MHD turbulence is generically strong in the sense that the waves which comprise it are critically damped. Nevertheless, deep inside the inertial range, turbulent fluctuations are small. Their energy density is less than that of the background field by a factor θ2(k⊥) « 1. MHD cascades are best understood geometrically. Wave packets suffer distortions as they move along magnetic field lines perturbed by counter propagating wave packets. Field lines perturbed by unidirectional waves map planes perpendicular to the local field into each other. Shear Alfvén waves are responsible for the mapping’s shear and slow waves for its dilatation. The former exceeds the latter by θ−1 (k⊥) » 1 which accounts for dominance of the shear Alfvén waves in controlling the cascade dynamics.

Keywords

MHDTurbulence
Type
Chapter One Introduction to Interstellar Scintillation
Information
International Astronomical Union Colloquium , Volume 182: Sources and Scintillations: Refraction and Scattering in Radio Astronomy , 2001 , pp. 17 - 23
Copyright
Copyright © Kluwer 2001

References

Goldreich, P. and Sridhar, S.: 1995, Toward a Theory of Interstellar Turbulence. II. Strong Alfvénic Turbulence, Astrophys. J. 438, 763–775.CrossRefGoogle Scholar
Goldreich, P. and Sridhar, S.: 1997, Magnetohydrodynamic Turbulence Revisited, Astrophys. J. 485, 680–688.Google Scholar
Iroshnikov, P.S.: 1963, Turbulence of a Conducting Fluid in a Strong Magnetic Field, Astronomicheskii Zhurnal 40, 742–750.Google Scholar
Kraichnan, R.H.: 1965, Inertial Range Spectrum of Hydromagnetic Turbulence, Physics of Fluids 8, 1385–1387.CrossRefGoogle Scholar
Ng, C.S. and Bhattacharjee, A.: 1996, Interaction of Shear-Alfven Wave Packets: Implication for Weak Magnetohydrodynamic Turbulence in Astrophysical Plasmas, Astrophys. J. 465, 845–854.Google Scholar
Shebalin, J.V., Matthaeus, W.H. and Montgomery, D.: 1983, Anisotropy in MHD Turbulence Due to a Mean Magnetic Field, J. Plasma Phys. 29, 525–547.Google Scholar