Article contents
Optimised uncertainty and cost operating characteristics: newtools for conformity assessment. Application to geometrical product control in automobileindustry
Published online by Cambridge University Press: 17 December 2010
Abstract
Translating measurement uncertainty into terms of effective impact associated withmanufacture, testing and incorrect assessment gives a more “stakeholder” motivated (andultimately optimised) approach to decision-making in conformance assessment. Recentlydeveloped decision-theory tools include the “optimized uncertainty” methodology and the“operating cost characteristic”. Overall costs, E, consisting of a sum oftesting costs, D, and the costs, C, associated withcustomer risk, can be calculated with the expression:
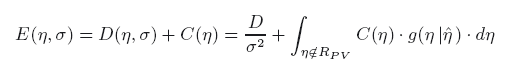
with 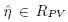 , whereRPV denotes the region of permissiblevalues and σ is a measure of dispersion. A complete, 3D surface ofoverall cost can indicate the optimum level of measurement effort of these two ranges, asrecently published by the author in a wide range of applications: optimized acceptancesampling; optimized testing of measurement instruments; and an analysis of optimisedcalibration intervals and “guard-banding”. This approach is illustrated in the presentwork for the example of geometrical product control in the car industry, specifically thegap in vehicle closure panels taking account of customer dissatisfaction.
, whereRPV denotes the region of permissiblevalues and σ is a measure of dispersion. A complete, 3D surface ofoverall cost can indicate the optimum level of measurement effort of these two ranges, asrecently published by the author in a wide range of applications: optimized acceptancesampling; optimized testing of measurement instruments; and an analysis of optimisedcalibration intervals and “guard-banding”. This approach is illustrated in the presentwork for the example of geometrical product control in the car industry, specifically thegap in vehicle closure panels taking account of customer dissatisfaction.
Information
- Type
- Research Article
- Information
- International Journal of Metrology and Quality Engineering , Volume 1 , Issue 2 , 2010 , pp. 105 - 110
- Copyright
- © EDP Sciences 2010
References
Références
- 8
- Cited by

