Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kessler, David A.
2008.
Epidemic Size in the SIS Model of Endemic Infections.
Journal of Applied Probability,
Vol. 45,
Issue. 03,
p.
757.
Kessler, David A
and
Shnerb, Nadav M
2008.
Novel exponents control the quasi-deterministic limit of the extinction transition.
Journal of Physics A: Mathematical and Theoretical,
Vol. 41,
Issue. 29,
p.
292003.
Lalley, Steven P.
2009.
Spatial epidemics: critical behavior in one dimension.
Probability Theory and Related Fields,
Vol. 144,
Issue. 3-4,
p.
429.
Greenwood, Priscilla E.
and
Gordillo, Luis F.
2009.
Mathematical and Statistical Estimation Approaches in Epidemiology.
p.
31.
Lalley, Steven P.
and
Zheng, Xinghua
2010.
Spatial epidemics and local times for critical branching random walks in dimensions 2 and 3.
Probability Theory and Related Fields,
Vol. 148,
Issue. 3-4,
p.
527.
Bhattacharya, Paritosh
Paul, Susmita
and
Choudhury, K. S.
2015.
Different Types of Epidemic Models and their Characteristic Behaviour by using Matlab.
Journal of Interdisciplinary Mathematics,
Vol. 18,
Issue. 5,
p.
569.
Simatos, Florian
2015.
State space collapse for critical multistage epidemics.
Advances in Applied Probability,
Vol. 47,
Issue. 3,
p.
715.
Crane, Harry
and
Lalley, Steven P.
2016.
Exchangeable Markov Processes on $$[k]^{\mathbb N}$$ [ k ] N with Cadlag Sample Paths.
Journal of Theoretical Probability,
Vol. 29,
Issue. 1,
p.
206.
Brightwell, Graham
House, Thomas
and
Luczak, Malwina
2018.
Extinction times in the subcritical stochastic SIS logistic epidemic.
Journal of Mathematical Biology,
Vol. 77,
Issue. 2,
p.
455.
Foxall, Eric
2021.
Extinction time of the logistic process.
Journal of Applied Probability,
Vol. 58,
Issue. 3,
p.
637.

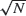 infected and
infected and  susceptible individuals, then when the time and the number currently infected are both scaled by
susceptible individuals, then when the time and the number currently infected are both scaled by 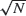 , the resulting process converges, as
, the resulting process converges, as 