Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Leorato, S.
Orsingher, E.
and
Scavino, M.
2003.
An alternating motion with stops and the related planar, cyclic motion with four directions.
Advances in Applied Probability,
Vol. 35,
Issue. 4,
p.
1153.
Bshouty, Daoud
Di Crescenzo, Antonio
Martinucci, Barbara
and
Zacks, Shelemyahu
2012.
Generalized Telegraph Process with Random Delays.
Journal of Applied Probability,
Vol. 49,
Issue. 03,
p.
850.
Bshouty, Daoud
Di Crescenzo, Antonio
Martinucci, Barbara
and
Zacks, Shelemyahu
2012.
Generalized Telegraph Process with Random Delays.
Journal of Applied Probability,
Vol. 49,
Issue. 3,
p.
850.

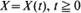 is examined. In particular, the third-order equations governing the distributions
is examined. In particular, the third-order equations governing the distributions 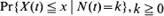 , (where
, (where  (where
(where 