Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kennedy, Douglas P.
1976.
Some martingales related to cumulative sum tests and single-server queues.
Stochastic Processes and their Applications,
Vol. 4,
Issue. 3,
p.
261.
Malyutin, A. A.
1988.
Distribution of a functional of continuous Markov processes.
Cybernetics,
Vol. 23,
Issue. 3,
p.
432.
Salminen, Paavo
and
Vallois, Pierre
2025.
Drawdowns of Diffusions.
ESAIM: Probability and Statistics,
Vol. 29,
Issue. ,
p.
357.

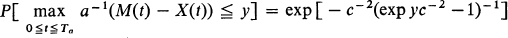
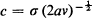 is the coefficient of variation of
is the coefficient of variation of 