Article contents
On the number of runs for Bernoulli arrays
Published online by Cambridge University Press: 14 July 2016
Abstract
We introduce and motivate the study of (n + 1) × r arrays X with Bernoulli entries X k,j and independently distributed rows. We study the distribution of 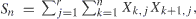 which denotes the number of consecutive pairs of successes (or runs of length 2) when reading the array down the columns and across the rows. With the case r = 1 having been studied by several authors, and permitting some initial inferences for the general case r > 1, we examine various distributional properties and representations of S n for the case r = 2, and, using a more explicit analysis, the case of multinomial and identically distributed rows. Applications are also given in cases where the array X arises from a Pólya sampling scheme.
which denotes the number of consecutive pairs of successes (or runs of length 2) when reading the array down the columns and across the rows. With the case r = 1 having been studied by several authors, and permitting some initial inferences for the general case r > 1, we examine various distributional properties and representations of S n for the case r = 2, and, using a more explicit analysis, the case of multinomial and identically distributed rows. Applications are also given in cases where the array X arises from a Pólya sampling scheme.
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Applied Probability Trust 2010
References
- 1
- Cited by

