Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Norros, I.
1995.
On the use of fractional Brownian motion in the theory of connectionless networks.
IEEE Journal on Selected Areas in Communications,
Vol. 13,
Issue. 6,
p.
953.
Hirchoren, G.A.
and
Arantes, D.S.
1998.
Predictors for the discrete time fractional Gaussian processes.
p.
49.
Breton, Alain Le
1998.
Une approche de type girsanov pour le filtrage dans un système linéaire simple avec bruit brownien fractionnaire.
Comptes Rendus de l'Académie des Sciences - Series I - Mathematics,
Vol. 326,
Issue. 8,
p.
997.
Le Breton, Alain
1998.
Filtering and parameter estimation in a simple linear system driven by a fractional Brownian motion.
Statistics & Probability Letters,
Vol. 38,
Issue. 3,
p.
263.
Duncan, T.E.
Hu, Y.Z.
and
Pasik-Duncan, B.
1999.
Some methods of stochastic calculus for fractional Brownian motion.
Vol. 3,
Issue. ,
p.
2390.
Ostring, S.A.M.
Sirisena, H.
and
Huson, I.
1999.
Dual dimensional ABR control scheme using predictive filtering of self-similar traffic.
p.
129.
Kleptsyna, M.L.
Le Breton, A.
and
Roubaud, M.-C.
2000.
Parameter Estimation and Optimal Filtering for Fractional Type Stochastic Systems.
Statistical Inference for Stochastic Processes,
Vol. 3,
Issue. 1-2,
p.
173.
Duncan, Tyrone E.
2000.
System Theory: Modeling, Analysis and Control.
Vol. 518,
Issue. ,
p.
97.
Östring, Sven A. M.
Sirisena, Harsha
and
Hudson, Irene
2000.
Networking 2000 Broadband Communications, High Performance Networking, and Performance of Communication Networks.
Vol. 1815,
Issue. ,
p.
36.
Duncan, Tyrone E.
Hu, Yaozhong
and
Pasik-Duncan, Bozenna
2000.
Stochastic Calculus for Fractional Brownian Motion I. Theory.
SIAM Journal on Control and Optimization,
Vol. 38,
Issue. 2,
p.
582.
Nuzman, Carl J.
and
Poor, H. Vincent
2000.
Linear estimation of self-similar processes via Lamperti's transformation.
Journal of Applied Probability,
Vol. 37,
Issue. 2,
p.
429.
Ribeiro, V.J.
Riedi, R.H.
Crouse, M.S.
and
Baraniuk, R.G.
2000.
Multiscale queuing analysis of long-range-dependent network traffic.
Vol. 2,
Issue. ,
p.
1026.
Hu, Yaozhong
2001.
Stochastics in Finite and Infinite Dimensions.
p.
153.
Ostring, S.A.M.
Sirisena, H.
and
Hudson, I.
2001.
Robust ABR control for uncertainties in long-range dependent traffic.
p.
53.
Thavaneswaran, A.
and
Heyde, C.C.
2001.
A note on filtering for long memory processes.
Mathematical and Computer Modelling,
Vol. 34,
Issue. 9-11,
p.
1139.
Tat-Keung Chan
Wing-Cheong Lau
and
Li, V.O.K.
2001.
A measurement-based congestion alarm for self-similar traffic.
Vol. 5,
Issue. ,
p.
1528.
Ostring, S.A.M.
Sirisena, H.R.
and
Hudson, I.
2001.
Rate control of elastic connections competing with long-range dependent network traffic.
IEEE Transactions on Communications,
Vol. 49,
Issue. 6,
p.
1092.
Ostring, S.A.M.
and
Sirisena, H.
2001.
The influence of long-range dependence on traffic prediction.
Vol. 4,
Issue. ,
p.
1000.
Brody, Dorje C
Syroka, Joanna
and
Zervos, Mihail
2002.
Dynamical pricing of weather derivatives.
Quantitative Finance,
Vol. 2,
Issue. 3,
p.
189.
Ahmed, N. U.
and
Charalambous, C. D.
2002.
Filtering for Linear Systems Driven by Fractional Brownian Motion.
SIAM Journal on Control and Optimization,
Vol. 41,
Issue. 1,
p.
313.

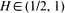 is discussed. The predictor
is discussed. The predictor 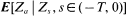 is represented as an integral with respect to
is represented as an integral with respect to 